Amity University Noida B.Tech Admissions 2025
Among Top 30 National Universities for Engineering (NIRF 2024) | 30+ Specializations | AI Powered Learning & State-of-the-Art Facilities
15 Questions around this concept.
A block of mass 10 kg starts sliding on a surface with an initial velocity of $9.8 \mathrm{~ms}^{-1}$. The coefficient of friction between the surface and block is 0.5. The distance covered by the block before coming to rest is: $\left[\right.$ use $\left.\mathrm{g}=9.8 \mathrm{~ms}^{-2}\right]$
A bag is gently dropped on a conveyor belt moving at a speed of $2 \mathrm{~m} / \mathrm{s}$. The coefficient of friction between the conveyor belt and bag is 0.4 . Initially, the bag slips on the belt before it stops due to friction. The distance travelled by the bag on the belt during slipping motion is: [Take $\mathrm{g}=10 \mathrm{~m} / \mathrm{s}^{-2}$ ]
A block of mass M slides down on a rough inclined plane with constant velocity. The angle made by the incline plane with horizontal is $\theta$. The magnitude of the contact force will be:
JEE Main 2026: Preparation Tips & Study Plan | Previous 10 Year Questions
JEE Main 2026: 100 Days Study Plan | High Scoring Chapters and Topics | Preparation Tips
JEE Main 2026: Top 30 Most Repeated Questions | Chapter Wise PYQs | Mock Test
JEE Main 2025 Most Scoring Concept: January Session | April Session
As shown in the figure a block is given an initial velocity of $20 \mathrm{~m} / \mathrm{s}$ in upward direction. What is the distance covered by the block to come to rest? $(g=10 \mathrm{~m} / \mathrm{s})$

A small ball of mass m starts at point A with speed vo and moves along a frictionless track AB as shown. The track BC has coefficient of friction $\mu$. The ball comes to a stop at C after traveling a distance L which is :

Three masses $\mathrm{M}=100 \mathrm{~kg}, \mathrm{~m}_1=10 \mathrm{~kg}$ and $\mathrm{m}_2=20 \mathrm{~kg}$ are arranged in a system as shown in figure. All the surfaces are frictionless and strings are inextensible and weightless. The pulleys are also weightless and frictionless. A force F is applied on the system so that the mass $\mathrm{m}_2$ moves upward with an acceleration of $2 \mathrm{~ms}^{-2}$. The value of F is : $\left(\right.$ Take $\left.\mathrm{g}=10 \mathrm{~ms}^{-2}\right)$

A cubical box of side 'a' sitting on a rough table top is pushed horizontally with a gradually increasing force until the box moves. If the force is applied at a height from the tabletop which is greater than a critical height H, the box topples first. If it is applied at a height less than H, the box starts sliding first. Then the coefficient of friction between the box and the tabletop is
Among Top 30 National Universities for Engineering (NIRF 2024) | 30+ Specializations | AI Powered Learning & State-of-the-Art Facilities
Campuses in Ropar, Agartala, Aizawl, Ajmer, Aurangabad, Calicut, Imphal, Itanagar, Kohima, Gorakhpur, Patna & Srinagar
A cubical box of side a sitting on a rough table-top is pushed horizontally with a gradually increasing force until the box moves. If the force is applied at a height from the tabletop which is greater than a critical height H,the box topples first. If it is applied at a height less than H, the box starts sliding first . Then the coefficient of friction between the box and the tabletop is
A box when dropped from a certain height reaches the ground with a speed $\nu$. When it slides from rest from the same height down a rough inclined plane inclined at an angle $45^{\circ}$ to the horizontal, it reaches the ground with a speed $\nu / 3$. The coefficient of sliding friction between the box and the plane is (acceleration due to gravity is $10 \mathrm{~ms}^{-2}$ )
A force of 100 N is just sufficient to pull a block of mass $10 \sqrt{3} \mathrm{~kg}$ on rough horizontal surface. What is angle friction? ( $\mathrm{g}=10 \mathrm{~m} / \mathrm{s}^2$ )
Case 1:- On the horizontal road
A block of mass m is moving initially with velocity u on a rough surface and due to friction, it comes to rest after covering a distance S.
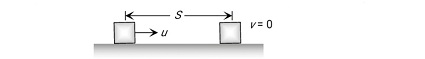

$
\begin{aligned}
& F=m a=\mu R \\
& m a=\mu m g \\
& a=\mu g \\
& V^2=u^2-2 a s \\
& S=\frac{u^2}{2 \mu g}=\frac{P^2}{2 \mu m^2 g} \\
& \mathrm{a}=\text { acceleration } \\
& \mu=\text { coefficient of friction } \\
& \mathrm{S}=\text { distance traveled } \\
& \mathrm{g}=\text { gravity } \\
& \mathrm{u}=\text { initial velocity } \\
& \mathrm{V}=\text { finally velocity } \\
& \mathrm{P}=\text { initial mometum=mu }
\end{aligned}
$
- Time taken to come to rest:
From equation $v=u-a t \Rightarrow 0=u-\mu g t \quad[$ As $v=0, a=\mu g]$
$
\therefore t=\frac{u}{\mu g}
$
- Force of friction acting on the body:
$
\begin{array}{ll}
F=m a & \\
F=m \frac{(v-u)}{t} & \\
F=\frac{m u}{t} & {[\text { As } v=0]} \\
F=\mu m g & {\left[\text { As } t=\frac{u}{\mu g}\right]}
\end{array}
$
Case 2:- On the inclined road :
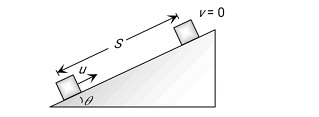

$\begin{aligned} & a=g[\sin \theta+\mu \cos \theta] \\ & V^2=u^2-2 a S \\ & 0=u^2-2 g[\sin \theta+\mu \cos \theta] S \\ & S=\frac{u^2}{2 g(\sin \theta+\mu \cos \theta)} \\ & \mathrm{S}=\text { distance traveled } \\ & \mu=\text { coefficient of friction } \\ & \mathrm{V}=\text { Final velocity } \\ & \mathrm{u}=\text { Initial velocity }\end{aligned}$
"Stay in the loop. Receive exam news, study resources, and expert advice!"
