Skew Hermitian Matrix - Practice Questions & MCQ

Skew-hermitian matrix

A square matrix $\mathrm{A}=\left[\mathrm{a}_{\mathrm{ij}}\right]_{\mathrm{n} \times \mathrm{n}}$ is said to be Skew-Hermitian matrix if $a_{i j}=-\overline{a_{i j}} \forall \mathrm{i}, \mathrm{j}_{\text {}}$
i.e. $\mathrm{A}^\theta=-\mathrm{A}, \quad\left[\right.$ where $\mathrm{A}^\theta$ is conjugate transpose of matrix A$]$

We know that when we take the transpose of a matrix, its diagonal elements remain the same, and while taking conjugate we just change sign from + ve to -ve $O R-v e$ to + ve in imaginary part of all elements, So to satisfy the condition $A$ ? $=-\mathrm{A}$, all diagonal element must be purely imaginary. As $A^?=-\mathrm{A}$ so
$\mathrm{a}_{\mathrm{ij}}=-\overline{\mathrm{a}_{\mathrm{ij}}} \forall \mathrm{i}, \mathrm{j}$
if we put $\mathrm{i}=\mathrm{j}$, we have

$
\mathrm{a}_{\mathrm{ii}}=-\overline{\mathrm{a}_{\mathrm{ii}}} \Rightarrow \mathrm{a}_{\mathrm{ii}}+\overline{\mathrm{a}_{\mathrm{ii}}}=0
$

$
\Rightarrow \mathrm{a}_{\mathrm{ii}}=0
$

Hence all diagonal element should be purely imaginary
E.g

Let, $\quad \mathrm{A}=\left[\begin{array}{ccc}-3 i & -3-4 i & -5+2 i \\ 3-4 i & 5 i & i \\ 5+2 i & i & 0\end{array}\right]$
Then,

$
\mathrm{A}^{\prime}=\left[\begin{array}{ccc}
-3 i & 3-4 i & 5+2 i \\
-3-4 i & 5 i & i \\
-5+2 i & i & 0
\end{array}\right]
$
 

Then,

$
\begin{aligned}
\mathrm{A}^{\prime} & =\left[\begin{array}{ccc}
-3 i & 3-4 i & 5+2 i \\
-3-4 i & 5 i & i \\
-5+2 i & i & 0
\end{array}\right] \\
\therefore \mathrm{A}^\theta & =\overline{\left(\mathrm{A}^{\prime}\right)}=\left[\begin{array}{ccc}
3 i & 3+4 i & 5-2 i \\
-3+4 i & -5 i & -i \\
-5-2 i & -i & 0
\end{array}\right] \\
& =-\left[\begin{array}{ccc}
-3 i & -3-4 i & -5+2 i \\
3-4 i & 5 i & i \\
5+2 i & i & 0
\end{array}\right]=-\mathrm{A}
\end{aligned}
$

here, A is Skew - Hermitian matrix as $\mathrm{A}^\theta=-\mathrm{A}$

Note:
1. for any square matrix $A$ with elements containing complex numbers, then $A-A$ ? is a skew hermitian matrix.

Proof: $\left(A-A^?\right)^?=A^?-\left(A^?\right)^?=A^?-A=-\left(A-A^?\right)$, hence skew-hermitian.
2. Every square matrix can be written as the sum of hermitian and skew-hermitian matrix i.e.

If $A$ is a square matrix, then we can write

$
A=\frac{1}{2}\left(A+A^\theta\right)+\frac{1}{2}\left(A-A^\theta\right)
$
 

Properties of hermitian and skew-hermitian matrices

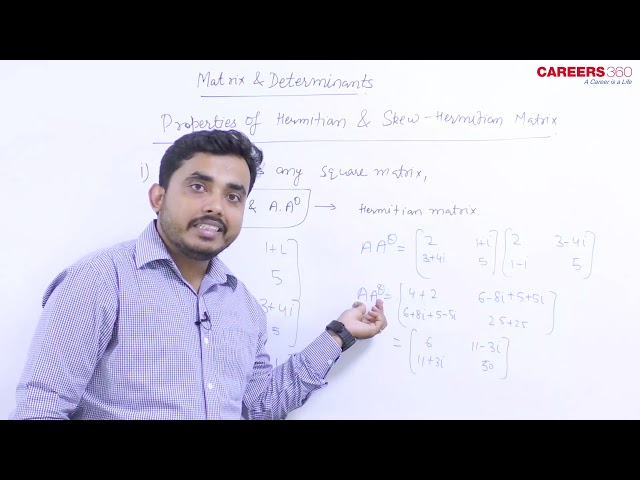
i) If A is a square matrix then AA^? and A^? A are hermitian matrix.

   Proof: for hermitian matrix A^? = A, so we check the condition on AA^? 

   (AA^?)^? = (A^?)^?A^? = AA^? hence it is hermitian, and in the same way, A^?A will also be hermitian.

ii) If A is hermitian matrix then:

    iA is a skew hermitian matrix, where i = √-1

    Proof: we need to show (iA)^? = -iA

    (iA)^?= A^?i^? = A^? (-i) = -iA^?

    Since A is hermitian so A^? = A

    Hence we have

    -iA^? = -iA. Proved.

iii) if A is a skew-hermitian matrix, then:

     iA is a hermitian matrix, where i = √-1

     Proof: we need to show (iA)? = iA

    (iA)^? = A^?i^? = A^?(-i) 

    A^?(-i) = Ai = iA   (since A is skew-hermitian, so A^? = -A)

iv) if A and B are hermitian matrices of the same order, then

  a.  cA and dB are also hermitian matrices of the same order when c and d are scalar real constant. 

       Since A and B are of the same order, hence they are conformable for addition and by multiplying through a scalar we         are just magnifying their values and nothing else, hence they will hold their property of hermitian matrices and cA +           dB will be a hermitian matrix.

  b.  AB is also hermitian if AB = BA

       Proof: (AB)^? = B^?A^? = BA = AB (Since A, B are hermitian so A^? = A, B^? =B)

  c.  AB + BA will also we hermitian

       Proof: from part (b) AB and BA is hermitian and from part (c) AB + BA will also be hermitian.

  d.  AB - BA will be skew-hermitian 

       Proof: we need to show (AB-BA)* = -(AB-BA)

       (AB-BA)* = (AB)* -  (BA)* = B*A* - A*B* = BA - AB = -(AB - BA) 

       Using A^? = A and B^? = B, proved.

v) if A and B are skew hermitian matrix then cA +dB will be skew-hermitian

    Proof are similar as above, just verify the basic condition, using the given condition of A and B.