Proof of the Vector Triple Product - Practice Questions & MCQ

For three vectors $\overrightarrow{\mathbf{a}}, \overrightarrow{\mathbf{b}}$ and $\overrightarrow{\mathbf{c}}$ vector triple product is defined as $\overrightarrow{\mathbf{a}} \times(\overrightarrow{\mathbf{b}} \times \overrightarrow{\mathbf{c}})$. $\vec{a} \times(\vec{b} \times \vec{c})=(\vec{a} \cdot \vec{c}) \cdot \vec{b}-(\vec{a} \cdot \vec{b}) \cdot \vec{c}$

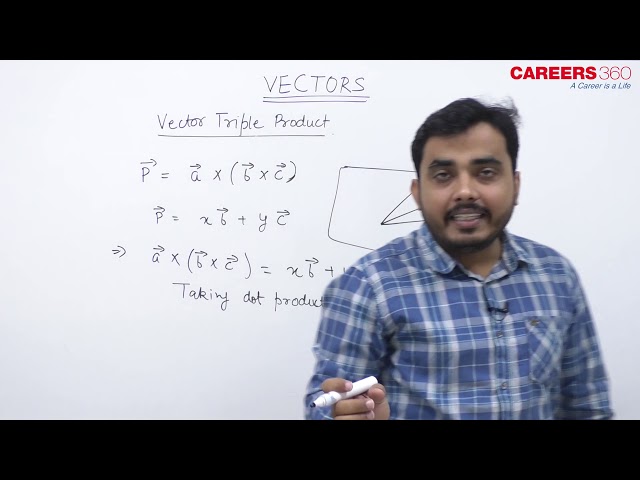
$\vec{p}=\vec{a} \times(\vec{b} \times \vec{c})$ is a vector perpendicular to $\vec{a}$ and $\vec{b} \times \vec{c}$, but $\vec{b} \times \vec{c}$ is a vector perpendicular to the plane of $\vec{b}$ and $\vec{c}$. Hence, vector $\vec{p}$ must lie in the plane of $\vec{b}$ and $\vec{c}$.
Let $\vec{p}=\vec{a} \times(\vec{b} \times \vec{c})=l \vec{b}+m \vec{c} \quad[l, m$ are scalars $]$
Taking the dot product of eq (i) with $\vec{a}$, we get
$
\vec{p} \cdot \vec{a}=l(\vec{a} \cdot \vec{b})+m(\vec{a} \cdot \vec{c})
$

$
\left[\begin{array}{l}
\because \vec{a} \times(\vec{b} \times \vec{c}) \text { is } \perp \vec{a} \\
\therefore \vec{a} \times(\vec{b} \times \vec{c}) \cdot \vec{a}=0
\end{array}\right]
$

Therefore,
$
\begin{array}{ll}
\Rightarrow & \vec{p} \cdot \vec{a}=0 \\
\Rightarrow & l(\vec{a} \cdot \vec{b})=-m(\vec{a} \cdot \vec{c}) \\
\Rightarrow & \frac{1}{\vec{a} \cdot \vec{c}}=\frac{-m}{\vec{a} \cdot \vec{b}}=\lambda \\
\Rightarrow & l=\lambda(\vec{a} \cdot \vec{c}) \\
\text { and } & m=-\lambda(\vec{a} \cdot \vec{b})
\end{array}
$

Substituting the value of $l$ and $m$ in Eq. (i), we get
$
\vec{a} \times(\vec{b} \times \vec{c})=\lambda[(\vec{a} \cdot \vec{c}) \vec{b}-(\vec{a} \cdot \vec{b}) \vec{c}]
$

Here, the value of $\lambda$ can be determined by taking specific values of $\vec{a}, \vec{b}$ and $\vec{c}$.

The simplest way to determine $\boldsymbol{\lambda}$ is by taking specific vectors $\vec{a}=\hat{i}, \vec{b}=\hat{i}, \vec{c}=\hat{j}$.
We have,
$
\begin{aligned}
& \vec{a} \times(\vec{b} \times \vec{c})=\lambda[(\vec{a} \cdot \vec{c}) \vec{b}-(\vec{a} \cdot \vec{b}) \vec{c}] \\
& \hat{i} \times(\hat{i} \times \hat{j})=\lambda[(\hat{i} \cdot \hat{j}) \hat{i}-(\hat{i} \cdot \hat{i}) \hat{j}] \\
& \hat{i} \times \hat{k}=\lambda[(0) \hat{i}-(1) \hat{j}] \Rightarrow-\hat{j}=-\lambda \hat{j} \\
\therefore \quad & \lambda=1
\end{aligned}
$

Hence,
$
\vec{a} \times(\vec{b} \times \vec{c})=(\vec{a} \cdot \vec{c}) \vec{b}-(\vec{a} \cdot \vec{b}) \vec{c}
$

NOTE:

1.
$
\begin{aligned}
& \overrightarrow{\mathbf{a}} \times(\vec{b} \times \vec{c})=(\vec{a} \cdot \vec{c}) \cdot \vec{b}-(\vec{a} \cdot \vec{b}) \cdot \vec{c} \\
& (\vec{a} \times \vec{b}) \times \vec{c}=(\vec{c} \cdot \overrightarrow{\mathbf{a}}) \cdot \vec{b}-(\vec{c} \cdot \vec{b}) \cdot \vec{a}
\end{aligned}
$
2. In general $\overrightarrow{\mathbf{a}} \times(\overrightarrow{\mathbf{b}} \times \overrightarrow{\mathbf{c}}) \neq(\overrightarrow{\mathbf{a}} \times \overrightarrow{\mathbf{b}}) \times \overrightarrow{\mathbf{c}}$ If $\overrightarrow{\mathbf{a}} \times(\overrightarrow{\mathbf{b}} \times \overrightarrow{\mathbf{c}})=(\overrightarrow{\mathbf{a}} \times \overrightarrow{\mathbf{b}}) \times \overrightarrow{\mathbf{c}}$ then the vectors $\vec{a}$ and $\vec{c}$ are collinear.