Linear Combination of Vectors - Practice Questions & MCQ

A vector $\vec{r}$ is said to be a linear combination of vectors $\vec{a}_1, \vec{a}_2, \vec{a}_3 \ldots \ldots, \vec{a}_n$ if there exist scalars $\lambda_1, \lambda_2, \lambda_3, \ldots \ldots, \lambda_n$ such that
$$
\vec{r}=\lambda_1 \vec{a}_1+\lambda_2 \vec{a}_2+\lambda_3 \vec{a}_3+\ldots \ldots+\lambda_n \vec{a}_n
$$

For example:
vectors $\vec{r}_1=\vec{a}+2 \vec{b}+3 \vec{c}$ and $\overrightarrow{r_2}=\vec{a}-4 \vec{b}-9 \vec{c}$ are linear combination of the vectors $\vec{a}, \vec{b}$ and $\vec{c}$.

Linear Independent Vectors
A system of vectors $\vec{a}_1, \vec{a}_2, \vec{a}_3 \ldots \ldots, \vec{a}_n$ is said to be linearly independent, if
$$
\begin{array}{ll} 
& \lambda_1 \vec{a}_1+\lambda_2 \vec{a}_2+\lambda_3 \vec{a}_3+\ldots \ldots+\lambda_n \vec{a}_n=0 \\
\Rightarrow \quad & \lambda_1=\lambda_2=\lambda_3=\ldots \ldots=\lambda_n=0
\end{array}
$$

It can be easily verified that

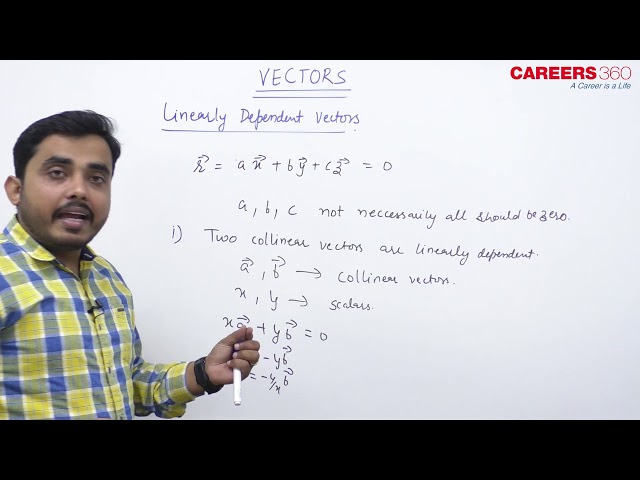
1. A pair of non-collinear vectors ( say a₁ and a₂ ) are linearly independent.

Let $\vec{a}_1$ and $\vec{a}_2$ are two non-collinear vectors such that $\lambda_1 \vec{a}_1+\lambda_2 \vec{a}_2=0$.
Let, $\quad \lambda_1, \lambda_2 \neq 0$
$$
\Rightarrow \quad \vec{a}_1=-\frac{\lambda_2}{\lambda_1} \vec{a}_2
$$

Now, $-\frac{\lambda_2}{\lambda_1}$ is a scalar, because $\lambda_1$ and $\lambda_2$ are scalars.
Hence, eq (i) expresses $\vec{a}_1$ as product of $\vec{a}_2$ by a scalar, so that $\vec{a}_1$ and $\vec{a}_2$ are collinear.
Which contradict the given fact because $\overrightarrow{a_1}$ and $\overrightarrow{a_2}$ are given to be non-collinear.
Th thus, our supposition that $\lambda_1 \neq 0$ and $\lambda_2 \neq 0$ is wrong.
Hence, $\lambda_1=0$ and $\lambda_2=0$

2. A triad of non-coplanar vector is linearly independent

If $\mathbf{a}, \mathbf{b}, \mathbf{c}$ are three non-zero, non-coplanar vectors and $x, y, z$ are three scalars such that
$$
x a+y b+z c=0
$$

Then, $x=y=z=0$
Proof: It is given that $\mathrm{xa}+\mathrm{yb}+\mathrm{zc}=0$
Suppose that $x \neq 0$
Then Eq. (i) can be written as
$$
\begin{aligned}
& x=-y \mathbf{b}-z \mathbf{c} \\
& \Rightarrow \mathbf{a}=-\frac{y}{x} \mathbf{b}-\frac{z}{x} \mathbf{c} \ldots(i i) \\
& \underline{y} \quad \underline{z}
\end{aligned}
$$

Now, $\bar{x}$ and $\frac{\sim}{x}$ are scalars because x , y and are scalars. Thus, Eq. (ii) expresses $\mathbf{a}$ as a linear combination of $\mathbf{b}$ and $\mathbf{c}$. Hence, $\mathbf{a}$ is coplanar with $\mathbf{b}$ and $\mathbf{c}$ which is contrary to our hypothesis because $\mathbf{a}, \mathbf{b}$ and $\mathbf{c}$ are given to be non-coplanar. Thus, our supposition that $x \neq 0$ is wrong.

Hence, x=0.

Similarly, we can prove that y=0 and z=0.

Note: 4 vectors are always linearly dependent

A system of vectors $\vec{a}_1, \vec{a}_2, \vec{a}_3 \ldots \ldots, \vec{a}_n$ is said to be linearly dependent if there exists scalars $\lambda_1, \lambda_2, \lambda_3, \ldots \ldots \ldots, \lambda_n$ not all zero such that
$$
\lambda_1 \vec{a}_1+\lambda_2 \vec{a}_2+\lambda_3 \vec{a}_3+\ldots \ldots+\lambda_n \vec{a}_n=0
$$

It can be easily verified that

1. A pair of collinear vectors is linearly dependent.

2. A triad of coplanar vectors is linearly dependent.

Test of collinearity of three points

Let $A(\vec{a}), B(\vec{b})$ and $C(\vec{c})$, be three points in space
If we can find $\mathrm{x}, \mathrm{y}, \mathrm{z}$, not all zero such that (i) $x \vec{a}+y \vec{b}+z \vec{c}=0$ and (ii) $x+y+z=0$

Then the points A, B, and C are collinear

Proof:

Let us suppose that points $A, B$ and $C$ are collinear and their position vectors are $\vec{a}, \vec{b}$ and $\vec{c}$ respectively. Let $C$ divide the join of $\vec{a}$ and $\vec{b}$ in the ratio $y: x$ Then,
or
$$
\begin{aligned}
& \vec{c}=\frac{x \vec{a}+y \vec{b}}{x+y} \\
& x \vec{a}+y \vec{b}-(x+y) \vec{c}=\overrightarrow{0} \\
& x \vec{a}+y \vec{b}+z \vec{c}=\overrightarrow{0}, \text { where } z=-(x+y) \\
& x+y+z=x+y-(x+y)=0
\end{aligned}
$$
or

Also,

Hence, the three points A, B, and C are collinear. 

Theorem 1:

If $\vec{a}$ and $\vec{b}$ are two non-zero, non-collinear vectors, then every vector $\vec{r}$ coplanar with $\vec{a}$ and $\vec{b}$ can be expressed in one and only one way as a linear combination $x \vec{a}+y \vec{b}$ where $x$ and $y$ being scalars.

Proof: 

Let $O$ be any point such that $\overrightarrow{O A}=\vec{a}$ and $\overrightarrow{O B}=\vec{b}$
As $\vec{r}$ is coplanar with $\vec{a}$ and $\vec{b}$, the lines $O A, O B$ and $O R$ are coplanar. Through $R$, draw lines parallel to $O A$ and $O B$, meeting them at $P$ and $Q$, respectively. Clearly,

Also,
$$
\begin{aligned}
\overrightarrow{O P} & =x \overrightarrow{O A}=x \vec{a} \quad(\because \overrightarrow{O P} \text { and } \overrightarrow{O A} \text { are collinear vectors }) \\
\overrightarrow{O Q} & =y \overrightarrow{O B}=y \vec{b} \quad(\because \overrightarrow{O Q} \text { and } \overrightarrow{O B} \text { are collinear vectors }) \\
\vec{r} & =\overrightarrow{O R}=\overrightarrow{O P}+\overrightarrow{P R}=\overrightarrow{O P}+\overrightarrow{O Q} \quad(\because \overrightarrow{O Q} \text { and } \overrightarrow{P R} \text { are equal }) \\
& =x \vec{a}+y \vec{b}
\end{aligned}
$$

Thus, $\vec{r}$ can be expressed in one way as a linear combination $x \vec{a}+y \vec{b}$.

Theorem 2

If $\vec{a}, \vec{b}$ and $\vec{c}$ are non-coplanar vectors, then any vector $\vec{r}$ can be uniquely expressed as a linear combination $\vec{x} \vec{a}+y \vec{b}+z \vec{c}$ where $x, y$ and $z$ being scalars.

NOTE:

1.

If vectors $\vec{a}=a_1 \hat{i}+a_2 \hat{j}+a_3 \hat{k}, \quad \vec{b}=b_1 \hat{i}+b_2 \hat{j}+b_3 \hat{k}$ and $\vec{c}=c_1 \hat{i}+c_2 \hat{j}+c_3 \hat{k}$ are coplanar, then
$$
\left|\begin{array}{lll}
a_1 & a_2 & a_3 \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|=0
$$
(Proof of this will be seen in the concept of Scalar triple Product)
2.

If vectors $x_1 \vec{a}+y_1 \vec{b}+z_1 \vec{c}, \quad x_2 \vec{a}+y_2 \vec{b}+z_2 \vec{c}$ and $x_3 \vec{a}+y_3 \vec{b}+z_3 \vec{c}$ are coplanar where $\vec{a}, \vec{b}$ and $\vec{c}$ are non-coplanar. Then,
$$
\left|\begin{array}{lll}
x_1 & y_1 & z_1 \\
x_2 & y_2 & z_2 \\
x_3 & y_3 & z_3
\end{array}\right|=0
$$