IIITDM Jabalpur JEE Main Cutoff 2025 - Check Previous Year Cutoff Here

3D Geometry - Practice Questions & MCQ

Edited By admin | Updated on Sep 18, 2023 18:34 AM | #JEE Main

Quick Facts

1 Questions around this concept.

Solve by difficulty

An arc PQ of a circle subtends a right angle at its centre O. The mid point of the arc PQ is R. If $\overrightarrow{\mathrm{OP}}=\overrightarrow{\mathrm{u}}, \overrightarrow{\mathrm{OR}}=\overrightarrow{\mathrm{v}}$ and $\overrightarrow{\mathrm{OQ}}=\alpha \overrightarrow{\mathrm{u}}+\beta \overrightarrow{\mathrm{v}},$ , then $\alpha ,\beta ^{2}$ are the roots of the equation :

$3x^{2}-2x-1=0$

$3x^{2} +2x-1=0$

$x^{2}-x-2=0$

$x^{2}+x-2=0$

View Solution

Concepts Covered - 1

Introduction to 3D Coordinate System

As we have learned, the two-dimensional rectangular coordinate system contains two perpendicular axes: the horizontal x-axis and the vertical y-axis. We can add a third dimension, the z-axis, which is perpendicular to both the x-axis and the y-axis. We call this system the three-dimensional rectangular coordinate system.

The system displayed follows the right-hand rule. If we take our right hand and align the fingers with the positive x-axis, then curl the fingers so they point in the direction of the positive y-axis, our thumb points in the direction of the positive z-axis. In this text, we always work with coordinate systems set up in accordance with the right-hand rule.

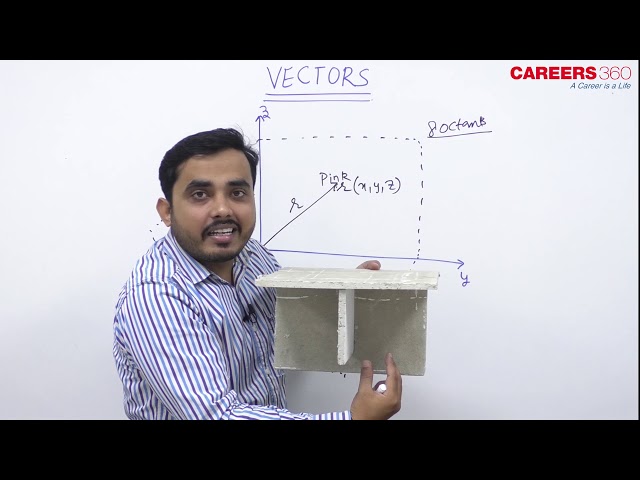
The three axes, X-axis, Y-axis and Z-axis are mutually perpendicular which defines three-dimensional (3D) coordinate system or space. Any point in this coordinate system has coordinates (x, y, z). Also, point in this system represents ordered triplets of set of cartesian product R x R x R Where, R is the set of real numbers.

If we take three axes, two at a time, then it will form three mutually perpendicular planes, XY-plane, YZ-plane and ZX-plane. This three plane divides space into eight regions called octants.

Coordinates of a Point in Space

Consider a point P(x, y, z) in three-dimensional system.

The x-coordinate of the point P is signed distance (OL) from the YZ-plane, i.e. a signed distance of P measured parallel to X-axis.

The y-coordinate of the point P is signed distance (ML) from the XZ-plane, i.e. a signed distance of P measured parallel to Y-axis.

The z-coordinate of the point P is signed distance (MP) from the XY-plane, i.e. a signed distance of P measured parallel to Z-axis.

The coordinates of the origin O are (0,0,0). The coordinates of any point on the x-axis will be as (x,0,0) and the coordinates of any point in the YZ-plane will be as (0, y, z).

The sign of the coordinates of a point determines the octant in which the point lies. The following table shows the signs of the coordinates in eight octants.

Octant	1^st	2^nd	3^rd	4^th	5^th	6^th	7^th	8^th
X	+	-	-	+	+	-	-	+
Y	+	+	-	-	+	+	-	-
Z	+	+	+	+	-	-	-	-

Distance between Two Points:

Let P(x₁ , y₁ , z₁ ) and Q ( x₂ , y₂ , z₂ ) be two points in a three-dimensional system of rectangular axes OX, OY and OZ.

Since, PAQ is right angled triangle with ∠PAQ as a right angle,

$\\\text{Using the Pythagorean theorem}\\\mathrm{\;\;\;\;\;\;\;\mathrm{\;\;\;\;\;\;\;\;\;\;PQ}^{2}=\mathrm{PA}^{2}+\mathrm{A} \mathrm{Q}^{2}\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\ldots(i)}\\\text{Also, triangle ANQ is right angle triangle with }\angle ANQ \text{ aright angle.}\\\mathrm{\therefore \;\;\;\;\;\;\;\;\;\;\;\;\;AQ^2=AN^2+QN^2\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\ldots(ii)}\\\text{from (i) and (ii)}\\\mathrm{\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;PQ^2=PA^2+AN^2+QN^2}\\\text{Now,}\\\mathrm{\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;PA}=y_{2}-y_{1}, \mathrm{AN}=x_{2}-x_{1} \text { and } \mathrm{NQ}=z_{2}-z_{1}\\\text{Hence,}\\\mathrm{\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;PQ}^{2}=\left(x_{2}-x_{1}\right)^{2}+\left(y_{2}-y_{1}\right)^{2}+\left(z_{2}-z_{1}\right)^{2}\\\\\therefore \;\;\;\;\;\;\;\;\;\;\mathrm{PQ}=\sqrt{\left(x_{2}-x_{1}\right)^{2}+\left(y_{2}-y_{1}\right)^{2}+\left(z_{2}-z_{1}\right)^{2}}$

This gives us the distance between two points P(x₁ , y₁ , z₁ ) and Q ( x₂ , y₂ , z₂ )

Distance of a point from the Axes and Origin

$\\\text{Distance of a point from }x-axis \mathrm{\;is\;\;\;\sqrt {y^2+z^2}.}\\\\\text{Distance of a point from }y-axis \mathrm{\;is\;\;\;\sqrt {x^2+z^2}.}\\\\\text{Distance of a point from }z-axis \mathrm{\;is\;\;\;\sqrt {y^2+x^2}.}$

Distance from Origin

$\\\text { If } x_{2}=y_{2}=z_{2}=0, \text { i.e., point } Q \text { is origin } O, \text { then } \mathrm{OP}=\sqrt{x_{1}^{2}+y_{1}^{2}+z_{1}^{2}}\\$