EASYUPES B.Tech Admissions 2025
Ranked #42 among Engineering colleges in India by NIRF | Highest Package 1.3 CR , 100% Placements | Last Date to Apply: 28th April
Motion Of Connected Blocks Over Pulley - Practice Questions & MCQ
Updated on Sep 18, 2023 18:34 AM | #JEE Main
Quick Facts
-
Motion of connected blocks over pulley is considered one the most difficult concept.
-
51 Questions around this concept.
Solve by difficulty
Two masses m1 = 5kg and m2 = 4.8 kg tied to a string are hanging over a light frictionless pulley. What is the acceleration (in m/s2 ) of the masses when lifted free to move?
( g = 9.8 m/s2 )
A light string passing over a smooth light pulley connects two blocks of masses m1 and m2 ( vertically) . If the acceleration of the system is g/8, then the ratio of the masses is
Two masses of 10 kg and 9 kg are connected by a string that passes over a smooth pulley; What is the tension in the string $\left(g=9.81 \mathrm{~ms}^{-2}\right)$
JEE Main 2025: Session 2 Result Out; Direct Link
JEE Main 2025: College Predictor | Marks vs Percentile vs Rank
New: JEE Seat Matrix- IITs, NITs, IIITs and GFTI | NITs Cutoff
Latest: Meet B.Tech expert in your city to shortlist colleges
Three masses each of 4 kg are connected by a string that passes through a smooth pulley; Tension between mass b and c?
Direction : In the following question , a statement of Assertion (A) is followed by a statement of reason (R) . Mark the correct choice as:
Assertion : The tension force on a body always act away from the body.
Reason : Tension force are always pushing force.
Calculate the acceleration of the system as shown in the figure, assume all the surfaces are smooth
Two blocks of masses 5kg and 10kg are connected to a pulley as shown in fig. What will be the acceleration if the pulley is set free?
What is the mechanical advantage of a single fixed pulley?
The system shown is released at rest. Speed of block A (in m/s), after B has descended by 2 cm is
JEE Main 2025 College Predictor
Know your college admission chances in NITs, IIITs and CFTIs, many States/ Institutes based on your JEE Main rank by using JEE Main 2025 College Predictor.
Use NowAs shown in the figure, a block of mass m = 200 g is suspended by a string. A force F is applied slowly at the lower end of the string.What will be the maximum value of F if bearing strength of the string is 5N?
Concepts Covered - 2
Motion of connected blocks over pulley
Equation of motion for $m_1$
$
F_{n e t}=T-m_1 g=m_1 a
$
Equation of Motion for $m_2$
$
\begin{aligned}
& F_{\text {net }}=m_2 g-T=m_2 a \\
& a=\frac{\left[m_2-m_1\right] g}{m_1+m_2} \\
& T=\frac{2 m_1 m_2 g}{m_1+m_2}
\end{aligned}
$
When one Block is hanging from a rope and one on the table
-
When one Block is hanging, other is on the Table
$\begin{aligned} a & =\frac{m_2 g}{m_1+m_2} \\ T & =\frac{m_1 m_2 g}{m_1+m_2}\end{aligned}$
-
Three blocks, two are hanging and one is at the rest on the smooth horizontal table
$
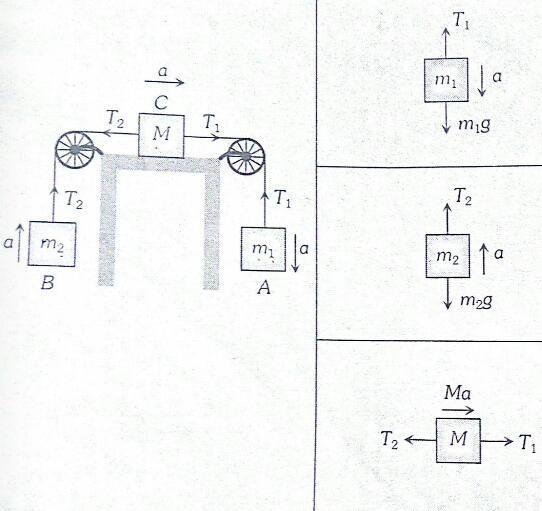
\begin{aligned}
& m_1 a=m_1 g-T_1 \\
& m_2 a=T_2-m_2 g \\
& T_1-T_2=M a
\end{aligned}
$
$
\begin{aligned}
& a=\frac{\left(m_1-m_2\right) g}{m_1+m_2+M} \\
& T_1=\frac{m_1\left(2 m_2+M\right) g}{\left(m_1+m_2+M\right)} \\
& T_2=\frac{m_2\left(2 m_1+M\right) g}{\left(m_1+m_2+M\right)}
\end{aligned}
$
Study it with Videos
Motion of connected blocks over pulley
When one Block is hanging from a rope and one on the table"Stay in the loop. Receive exam news, study resources, and expert advice!"
Get Answer to all your questions
JEE Main Articles
Back to top