Maximum and Minimum value of Trigonometric Function - Practice Questions & MCQ

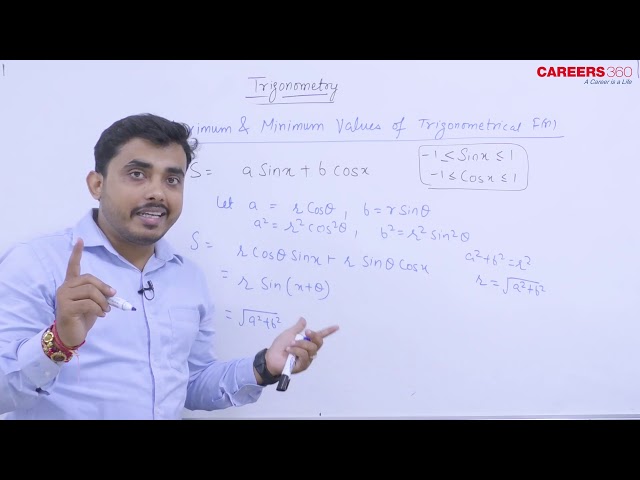
Maximum and Minimum Value of Trigonometric Function
We know that range of $\sin x$ and $\cos x$ which is $[-1,1]$,
If there is a trigonometric function in the form of $a \sin x+b \cos x$, then replace a with $r \cos \theta$ and $b$ with $\mathrm{r} \sin \theta$.

Then we have,

$
\begin{aligned}
a \sin \mathrm{x}+b \cos \mathrm{x} & =r \cos \theta \sin \mathrm{x}+r \sin \theta \cos \mathrm{x} \\
& =r(\cos \theta \sin \mathrm{x}+\sin \theta \cos \mathrm{x}) \\
& =r \sin (\mathrm{x}+\theta)
\end{aligned}
$

where $r=\sqrt{a^2+b^2}$ and, $\tan \theta=\frac{b}{a}$
Since, $\quad-1 \leq \sin (x+\theta) \leq 1$
Multiply with 'r'

$
\begin{aligned}
& \Rightarrow-\mathrm{r} \leq \mathrm{r} \sin (\mathrm{x}+\theta) \leq \mathrm{r} \\
& \Rightarrow-\sqrt{a^2+b^2} \leq \mathrm{r} \sin (\mathrm{x}+\theta) \leq \sqrt{a^2+b^2} \\
& \Rightarrow-\sqrt{a^2+b^2} \leq a \sin \mathrm{x}+b \cos \mathrm{x} \leq \sqrt{a^2+b^2}
\end{aligned}
$
So, the minimum value of trigonometric function a $\sin \mathrm{x}+\mathrm{b} \cos \mathrm{x}$ is $-\sqrt{a^2+b^2}$ and maximum value is $\sqrt{a^2+b^2}$.