Trigonometric Inequality - Practice Questions & MCQ

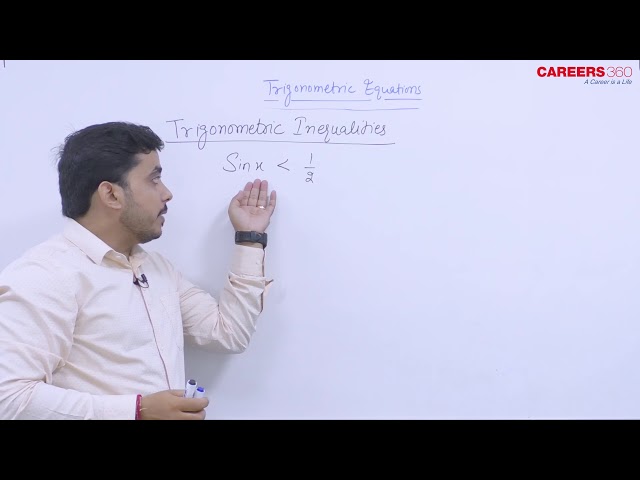
Trigonometric Inequality

The trigonometric inequation is of the type $f(x) \geq a$ or $f(x) \leq a$, where $f(x)$ is some trigonometric ratio.
The following steps should be taken to solve such types of inequations
Draw the graph of $f(x)$ in an interval length equal to the fundamental period of $f(x)$.
Draw the line $\mathrm{y}=\mathrm{a}$.
Take the portion of the graph for which the inequation is satisfied.
To generalize, add $n T(n \in I)$, where $T$ is the fundamental period of $f(x)$.
Example
What is the solution set of inequality $\cos x>1 / 2 ?$
Solution
1. Fundamental period of $\cos (x)$ is $2 \pi$, so we draw its graph in any interval of length $2 \pi$. Here we are drawing the graph in $-\pi$ to $\pi$
2. When $\cos x=1 / 2$, then two values of $x$ between $-\pi$ and $\pi$ is $-\pi / 3$ and $\pi / 3$
3. From the graph

4. $\cos x>1 / 2$ for $-\pi / 3<x<\pi / 3$

Now the same interval will repeat in every period of length $2 \pi$
Hence, $\cos x>1 / 2$ when $-\pi / 3+2 n \pi<x<\pi / 3+2 n \pi, n \in I$
Note: We can also interval from 0 to $2 \pi$ instead of $-\pi$ to $\pi$