Thermal Expansion In Liquids And Gases - Practice Questions & MCQ

Thermal Expansion in Liquids

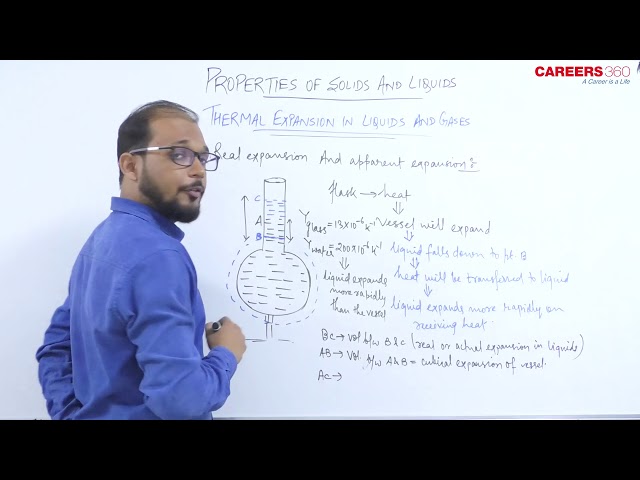
• Like solids, liquids do not have linear and superficial expansion but liquid only undergoes volume expansion.
• We always need some solid vessel to keep the liquid, so liquids are always to be heated along with a vessel which contains them so initially on heating the system (System is liquid + vessel here). Initially, the level of liquid in the vessel falls (vessel expands more since it absorbs heat and liquid expands less) as the volume expansion co-efficient of solid is more than that of liquid but later on, it starts rising due to faster expansion of the liquid (because now solid transfer all the heat to liquid and that is the condition of steady-state)

So, from above we can conclude that the actual increase in the volume of the liquid = The apparent increase in the volume of liquid + the increase in the volume of the vessel.

Basically, liquids have two coefficients of volume expansion - 

1. Co-efficient of apparent expansion : It is due to an apparent (Apparent means that appears but not real) increase in the volume of liquid. This happens when the expansion of the vessel containing the liquid is not taken into account.

$
\gamma_a=\frac{\text { Apparent expansion in volume }}{\text { Initial vdume } \times \Delta \theta}=\frac{(\Delta V)_o}{V \times \Delta \theta}
$

2. Co-efficient of real expansion $\gamma_r$ : It is due to the actual increase in the volume of liquid due to heating. In this expansion of vessel containing the liquid is taken into account.

$
\gamma_r=\frac{\text { Real increase in volume }}{\text { Initial vdume } \times \Delta \theta}=\frac{(\Delta V)}{V \times \Delta \theta}
$

Also coefficient of expansion of flask $\gamma_{\text {vessel }}=\frac{\Delta V_{\text {vessel }}}{V \times \Delta \theta}$
So, $\gamma_{\text {Real }}=\gamma_{\text {Apporent }}+\gamma_{\text {Vessel }}$
So the change (apparent change) in volume in liquid relative to the vessel is -

$
\Delta V_{a p p}=V \gamma_{\text {app }} \Delta \theta=V\left(\gamma_{\text {Real }}-\gamma_{\text {Vessel }}\right) \Delta \theta=V\left(\gamma_r-3 \alpha\right) \Delta \theta
$

Where, $\alpha=$ Coefficient of linear expansion of the vessel.

Anomalous expansion of water: Generally any material expands on heating and contracts on cooling. But in the case of water, it expands on heating if its temperature is greater than 4°C. In the range 0°C to 4°C, water contracts on heating and expands on cooling, i.e.  is negative. So water has this special property, which is not found in any existing natural material. This behaviour of water in the range from 0°C to 4°C is called anomalous expansion. You can see it with the help of a graph.

This is the anomalous behaviour of water which causes ice to form first at the surface of a lake in cold weather. So, as winter approaches, the water temperature increases initially at the surface. It results in the water sinking because of its increased density. Consequently, the surface reaches 0°C first and because of that the lake becomes covered with ice. This property of water makes the aquatic life survive the cold winter as the lake bottom remains unfrozen at a temperature of about 4°C.

At 4°C, density of water is maximum while its specific volume is minimum.

Variation of Density with Temperature -

Most substances (solid and liquid) expand heat is supplied to them, i.e., the volume of a given mass of a substance increases on heating, so the density should decrease $\left(\right.$ as $\left.\rho \propto \frac{1}{V}\right)$ cooling as follows -

$
\begin{aligned}
& \quad \frac{\rho^{\prime}}{\rho}=\frac{V}{V^{\prime \prime}}=\frac{V}{V+\Delta V}=\frac{V}{V+\gamma V \Delta \theta}=\frac{1}{1+\gamma \Delta \theta} \\
& \quad \Rightarrow \rho^{\prime}=\frac{\rho}{1+\gamma \Delta \theta}=\rho(1+\gamma \Delta \theta)^{-1}=\rho(1-\gamma \Delta \theta)
\end{aligned}
$

It means that the density is inversely proportional to the volume. From that, we can deduce the expression of density after heating or

Here, $\rho$ and $\rho^{\prime}$ is the density before and after heating the material

Expansion of Gases -
As we know that the gases have no definite shape. It takes the shape of the vessel in which it is kept. Therefore gases have only volume expansion. Since the expansion of the container (Because the container is solid) is negligible in comparison to the gases, therefore gases have only real expansion.
(1) Coefficient of volume expansion: At constant pressure, the unit volume of a given mass of a gas, increases with a $1^{\circ} \mathrm{C}$ rise of temperature, which is called the coefficient of volume expansion.

$
\alpha=\frac{\Delta V}{V_0} \times \frac{1}{\Delta \theta} \Rightarrow \text { Final volume } V^{\prime}=V(1+\alpha \Delta \theta)
$

(2) Coefficient of pressure expansion:

$
\beta=\frac{\Delta P}{P} \times \frac{1}{\Delta \theta}
$

$\therefore$ Final pressure $P^{\prime}=P(1+\beta \Delta \theta)$