Variation Of Pressure In An Accelerated Fluid - Practice Questions & MCQ

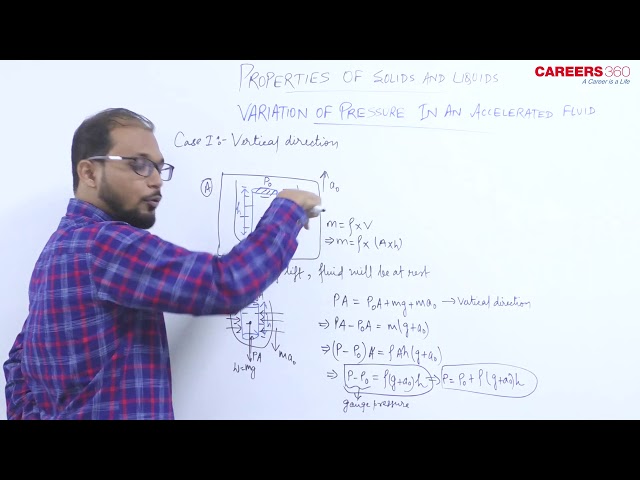
 Case I-  When Acceleration in the vertical direction

1. When the liquid container is moving with constant acceleration in an upward direction

         Consider a cylindrical element of height h and Area A as shown in the below figure.

The force on the top face of the element $=P_1 A$
The force on the bottom face of the element $=P_2 A$
If $a$ is the acceleration of the liquid then
We can write

$
P_2 A-\left(h A \rho g+P_1 A\right)=m a
$

Where $m$ is the mass of the element of the liquid and which is given by

$
m=\rho h A
$

Where $\rho=$ density of liquid
So using this we get

$
P_2-P_1=\rho(g+a) h=\rho g_{e f f} h
$

2. When the liquid container is moving with constant acceleration in a downward direction
I. constant downward acceleration $(\mathrm{a}<\mathrm{g})$

So $g_{\text {eff }}$ for the below figure is given by $g_{\text {eff }}=(g-a)$

     

And Pressure at point A is given as

$
P=\rho(g-a) h=\rho g_{e f f} h
$
 

              II.  constant  downward acceleration (a=g)

                  The pressure became zero everywhere when a=g

                III.   constant  downward acceleration (a> g)

                        In this case, the fluid occupies the upper part of the container as shown in the figure.

 

 Case II-  When Acceleration in Horizontal  direction

If a liquid in the tank is moving on a horizontal surface with some constant acceleration a

Then the free surface of the liquid takes the shape as shown by the dotted line in the figure.

Now consider a cylindrical element of length l and cross-section area A

So the force on the left face of the cylinder is $F_1=P_1 A$
While force on the right face of the cylinder is $F_2=P_2 A$
And we can also write

$
P_1=\rho g y_1 \text { and } P_2=\rho g y_2
$

And the mass of the element of the liquid and which is given by

$
m=\rho l A
$

Where $\rho=$ density of liquid
So using Newton's second law for the element

$
\begin{gathered}
F_1-F_2=m a \\
\text { or } P_1 A-P_2 A=m a \\
\text { or }\left(\rho g y_1-\rho g y_2\right) A=A l \rho a \\
\text { or } \frac{y_1-y_2}{l}=\frac{a}{g}=\tan \theta
\end{gathered}
$
 

So we can say that

 The free surface of the liquid makes an angle $\theta$ with horizontal 

Or the free surface of the liquid orient itself perpendicular to the direction of net effective gravity.

So for the below figure, we can say that

Pressure will vary in the horizontal direction.

And the Pressure gradient in the x-direction is given as

$\frac{d p}{d x}=-\rho a_x$

Where -ve sign indicates pressure increases in a direction opposite to the direction of acceleration.