MEDIUMUPES B.Tech Admissions 2025
ApplyRanked #42 among Engineering colleges in India by NIRF | Highest Package 1.3 CR , 100% Placements | Last Date to Apply: 28th April
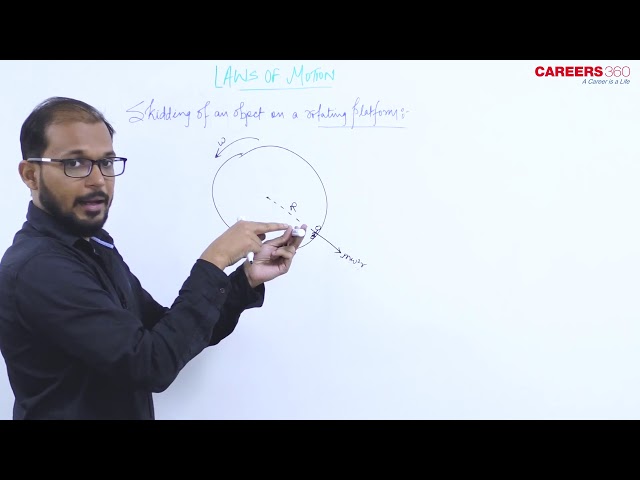
Skidding Of Object On A Rotating Platform - Practice Questions & MCQ
Edited By admin | Updated on Sep 18, 2023 18:34 AM | #JEE Main
Quick Facts
-
4 Questions around this concept.
Solve by difficulty
A block of mass $m$ is placed on a rotating platform at a distance r from the axis of rotation. What should be the maximum angular velocity to avoid skidding of the block [ take $\mu=$ the coefficient between the block and rotating platform]
A block of mass m is kept on the edge of the horizontal turn table of radius R
Turn table is rotating with constant angular velocity w . coefficient of friction is . If the block is
just about to move find angular velocity w of the turn table
Concepts Covered - 1
Skidding of object on a Rotating Platform
Centrifugal force $\leq$ Force of friction
$m \omega^2 r \leq \mu m g$
$\therefore \omega_{\max }=\sqrt{\frac{\mu g}{r}}=$ lt is the maximum angular velocity of rotation of the platform, so that object will not skid on it.
$\omega=$ Angular velocity
$r=$ radius
$\mu=$ coefficient of friction
Study it with Videos
Skidding of object on a Rotating Platform"Stay in the loop. Receive exam news, study resources, and expert advice!"
Get Answer to all your questions
JEE Main Articles
Apr 22, 2025
Back to top