Semiperimeter and Half Angle Formulae - Practice Questions & MCQ

Half-Angle Formula for Sine

$
\begin{aligned}
& \sin \frac{A}{2}=\sqrt{\frac{(s-b)(s-c)}{b c}} \\
& \sin \frac{B}{2}=\sqrt{\frac{(s-a)(s-c)}{a c}} \\
& \sin \frac{C}{2}=\sqrt{\frac{(s-a)(s-b)}{a b}}
\end{aligned}
$
We know that,

$
\cos \mathrm{A}=1-2 \sin ^2 \frac{\mathrm{~A}}{2} \Rightarrow \sin ^2 \frac{\mathrm{~A}}{2}=\frac{1-\cos \mathrm{A}}{2}
$
Now, for any $\triangle A B C$

$
\cos \mathrm{A}=\frac{\mathrm{b}^2+\mathrm{c}^2-\mathrm{a}^2}{2 \mathrm{bc}}
$

Using the above two formulas

$
\begin{aligned}
\begin{aligned}
\sin ^2 \frac{A}{2} & =\frac{1}{2}\left[1-\frac{b^2+c^2-a^2}{2 b c}\right] \\
& =\frac{1}{2}\left[\frac{2 \mathrm{bc}-\mathrm{b}^2-\mathrm{c}^2+\mathrm{a}^2}{2 \mathrm{bc}}\right] \\
& =\frac{1}{2}\left[\frac{\mathrm{a}^2-(\mathrm{b}-\mathrm{c})^2}{2 \mathrm{bc}}\right] \\
& =\frac{(\mathrm{a}-\mathrm{b}+\mathrm{c})(\mathrm{a}+\mathrm{b}-\mathrm{c})}{4 \mathrm{bc}} \\
& =\frac{(2 \mathrm{~s}-2 \mathrm{~b})(2 \mathrm{~s}-2 \mathrm{c})}{4 \mathrm{bc}} \\
\Rightarrow \sin ^2 \frac{\mathrm{~A}}{2} & =\frac{(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}{\mathrm{bc}} \\
\text { As } 0 & <\frac{\mathrm{A}}{2}<\frac{\pi}{2}, \mathrm{so} \sin \frac{\mathrm{~A}}{2} \\
& \\
\sin \frac{\mathrm{~A}}{2} & =\sqrt{\frac{(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}{\mathrm{bc}}}
\end{aligned} \text {. }
\end{aligned}
$
Similarly, we can derive other formulas.

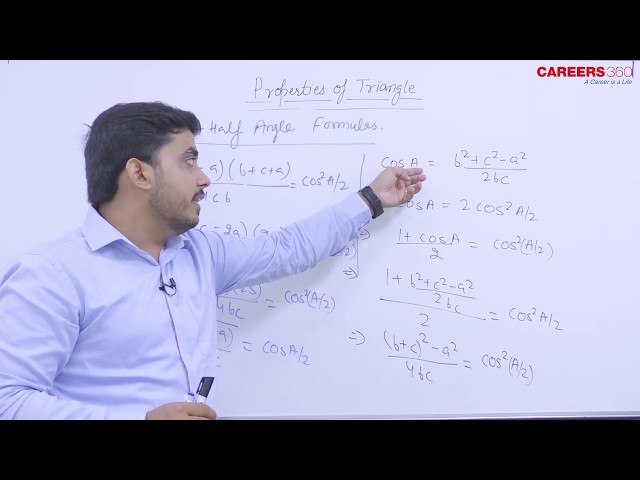
Half-Angle Formula for Cosine and Tangent

$
\begin{aligned}
\cos \frac{A}{2} & =\sqrt{\frac{(s)(s-a)}{b c}} \\
\cos \frac{B}{2} & =\sqrt{\frac{(s)(s-b)}{a c}} \\
\cos \frac{C}{2} & =\sqrt{\frac{(s)(s-c)}{a b}}
\end{aligned}
$
Proof:
We know that

$
\cos \mathrm{A}=2 \cos ^2 \frac{\mathrm{~A}}{2}-1 \Rightarrow \cos ^2 \frac{\mathrm{~A}}{2}=\frac{1+\cos \mathrm{A}}{2}
$
And for any $\triangle A B C$

$
\cos \mathrm{A}=\frac{\mathrm{b}^2+\mathrm{c}^2-\mathrm{a}^2}{2 \mathrm{bc}}
$

Using the above two formulas

$
\begin{aligned}
\cos ^2 \frac{A}{2} & =\frac{1}{2}\left[1+\frac{b^2+c^2-a^2}{2 b c}\right] \\
& =\frac{1}{2}\left[\frac{\left.2 \mathrm{bc}+\mathrm{b}^2+\mathrm{c}^2-\mathrm{a}^2\right]}{2 \mathrm{bc}}\right] \\
& =\frac{1}{2}\left[\frac{\left.(\mathrm{~b}+\mathrm{c})^2-\mathrm{a}^2\right]}{2 \mathrm{bc}}\right] \\
& =\frac{(\mathrm{b}+\mathrm{c}+\mathrm{a})(\mathrm{b}+\mathrm{c}-\mathrm{a})}{4 \mathrm{bc}} \\
& =\frac{(\mathrm{b}+\mathrm{c}+\mathrm{a})(\mathrm{a}+\mathrm{b}+\mathrm{c}-2 \mathrm{a})}{4 \mathrm{bc}} \\
& =\frac{(2 \mathrm{~s})(2 \mathrm{~s}-2 \mathrm{a})}{4 \mathrm{bc}} \\
\Rightarrow \cos ^2 \frac{\mathrm{~A}}{2} & =\frac{(\mathrm{s})(\mathrm{s}-\mathrm{a})}{\mathrm{bc}} \\
\text { As } 0 & <\frac{\mathrm{A}}{2}<\frac{\pi}{2}, \mathrm{so}+\mathrm{b}+\mathrm{cos} \frac{\mathrm{~A}}{2}>0 \\
\cos \frac{\mathrm{~A}}{2} & =\sqrt{\frac{(\mathrm{s})(\mathrm{s}-\mathrm{a})}{\mathrm{bc}}}
\end{aligned}
$
Similarly, we can derive other formulas.

Half Angle Formula for tan

$
\begin{aligned}
& \tan \frac{A}{2}=\sqrt{\frac{(s-b)(s-c)}{s(s-a)}} \\
& \tan \frac{B}{2}=\sqrt{\frac{(s-a)(s-c)}{s(s-b)}} \\
& \tan \frac{C}{2}=\sqrt{\frac{(s-a)(s-b)}{s(s-c)}}
\end{aligned}
$
This half-angle formula can be proved using $\tan x=\sin x / \cos x$, and using the half-angle formula of sine and cosine.