Tangents to Hyperbolas MCQ - Practice Questions & Answers

NIRF Ranking 2024 (Out) - List of Top Engineering Colleges in India

Quick Facts

Equation of Tangent of Hyperbola in Point Form, Equation of Tangent of Hyperbola in Parametric Form and Slope Form is considered one of the most asked concept.
63 Questions around this concept.

Solve by difficulty

The tangent at a point P on the hyperbola $\mathrm{\frac{x^2}{a^2}-\frac{y^2}{b^2}=1}$ meets one of the directrices in F. If PF subtends
an angle $\mathrm{\theta }$ at the corresponding focus, then $\mathrm{\theta }$ equals

$\mathrm{\pi / 4}$

$\mathrm{\pi / 2}$

$\mathrm{3\pi / 4}$

$\mathrm{\pi }$

View Solution

Tangents are drawn to $\mathrm{3 x^2-2 y^2=6}$ from a point P. If these tangents intersect the coordinate axes at concyclic points, The locus of P is

$\mathrm{\frac{1}{x^2}+\frac{1}{y^2}=5}$

$\mathrm{x^2-y^2=5}$

$\mathrm{\frac{1}{x^2}-\frac{1}{y^2}=5}$

View Solution

Concepts Covered - 0

Equation of Tangent of Hyperbola in Point Form

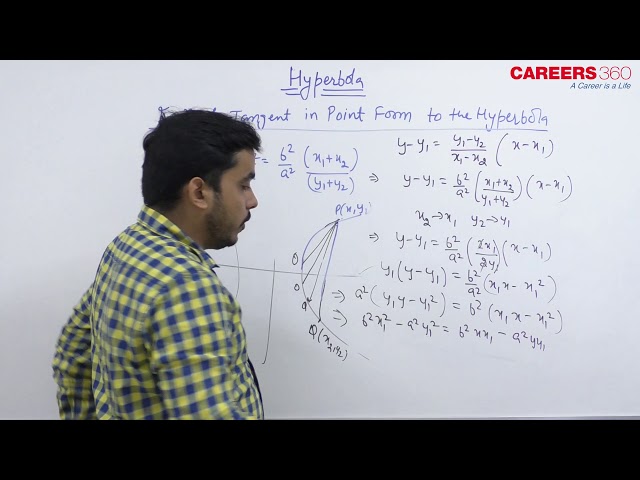
Equation of Tangent of Hyperbola in Point Form:

$\\ {\text {The equation of tangent to the hyperbola, } \frac{\mathrm{x}^{2}}{\mathrm{a}^{2}}-\frac{\mathrm{y}^{2}}{\mathrm{b}^{2}}=1 \text { at point }\left(\mathrm{x}_{1}, \mathrm{y}_{1}\right) \text { is }} \\ {\frac{\mathrm{x} \mathrm{x}_{1}}{\mathrm{a}^{2}}-\frac{\mathrm{y} \mathrm{y}_{1}}{\mathrm{b}^{2}}=1}$

$\\ {\text { Differentiating } \frac{x^{2}}{a^{2}}-\frac{y^{2}}{b^{2}}=1 \text { w.r.t. } x, \text { we have }} \\\\ {\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\frac{2 x}{a^{2}}-\frac{2 y}{b^{2}} \frac{d y}{d x}=0}\\\\ {\Rightarrow \quad \;\;\;\;\;\;\;\;\frac{d y}{d x}=\frac{b^{2} x}{a^{2} y}} \\\\ {\Rightarrow \quad\;\;\;\;\;\;\;\left(\frac{d y}{d x}\right)_{(x, y)}=\frac{b^{2} x_{1}}{a^{2} y_{1}}} \\\\ \text { Hence, equation of the tangent is } y-y_{1}=\frac{b^{2} x_{1}}{a^{2} y_{1}}\left(x-x_{1}\right) \\\\ \mathrm{or\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\frac{x x_{1}}{a^{2}}-\frac{y y_{1}}{b^{2}}=\frac{x_{1}^{2}}{a^{2}}-\frac{y_{1}^{2}}{b^{2}}}\\\\ \text { But }\left(x_{1}, y_{1}\right) \text { lies on the hyperbola } \Rightarrow \frac{x_{1}^{2}}{a^{2}}-\frac{y_{1}^{2}}{b^{2}}=1$

$\\ {\text { Hence, equation of the tangent is }} \\\\ {\;\;\;\;\;\;\;\;\;\;\;\frac{x x_{1}}{a^{2}}-\frac{y y_{1}}{b^{2}}=1}\\\\ \text { or } \quad \frac{x x_{1}}{a^{2}}-\frac{y y_{1}}{b^{2}}-1=0 \text { or } T=0\\\\ \text { where } \quad T=\frac{x x_{1}}{a^{2}}-\frac{y y_{1}}{b^{2}}-1$

Note:

T = 0 can be used to get the equation of tangent on the point (x₁, y₁) lying on any general hyperbola as well.

Equation of Tangent of Hyperbola in Parametric Form and Slope Form

Equation of Tangent of Hyperbola in Parametric Form and Slope Form

Parametric Form
$\\ {\text {The equation of tangent to the hyperbola, } \frac{\mathrm{x}^{2}}{\mathrm{a}^{2}}-\frac{\mathrm{y}^{2}}{\mathrm{b}^{2}}=1 \text { at }(\mathrm{a} \sec \theta, \mathrm{b} \tan \theta) \text { is }} \\ {\frac{\mathrm{x}}{\mathrm{a}} \sec \theta-\frac{\mathrm{y}}{\mathrm{b}} \tan \theta=1}$

(This can easily be derived by putting x₁ = a sec $\theta$ and y₁ = b tan $\theta$ in the point form of tangent)

Slope Form

We have studied that if the line y = mx + c is tangent to the hyperbola $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$ , then c² = a²m² - b². So the equation of tangent is $y=mx\pm\sqrt{a^2m^2-b^2}$ .

These equations are equations of two parallel tangents to hyperbola having slope m.

Study other Related Concepts

Tangents to Hyperbolas Current Topic

Coordinate Axes

Distance Between Two Points Formula

Section Formula & Conic Sections

Centroid, Incentre and Circumcentre and Orthocentre of a Triangle

Excenters of Triangle

Circumcentre and Orthocentre

Incentre

Area of Triangle in Coordinate Geometry

Locus

Transformations of Axes

"Stay in the loop. Receive exam news, study resources, and expert advice!"

Get Answer to all your questions

JEE Main Articles

UP BTech Admission 2024: Round 2 Choice Filling (Started), Seat Allotment, Merit List, Counselling, Process

Sep 07, 2024

Top Engineering Exams for NIOS Candidates

Sep 07, 2024

NIRF Ranking 2024 (Out) - List of Top Engineering Colleges in India

Sep 06, 2024

NIT Warangal Cutoff JEE Main 2024 - Check here

Sep 06, 2024

JEE Main 2025 Syllabus PDF - Subject-Wise Detailed Syllabus

Sep 06, 2024

Architecture Courses After 12th - Fees, Eligibility, Duration, Top Colleges

Sep 06, 2024

JEE Main Exam Pattern 2025 - Total Marks in JEE Mains, Paper Pattern, Duration

Sep 06, 2024

Top 10 Engineering Entrance Exams in India 2025

Sep 05, 2024

B.Tech/B.Arch Admissions OPEN

NIELIT University(Govt. of India Institution) B.Tech/ M.Tech

Apply

Last Date to Apply : 15th September | Campuses in Agartala, Aizawl, Ajmer, Aurangabad, Calicut, Imphal, Itanagar, Kohima, Gorakhpur, Patna & Srinagar

Amity University, Noida B.Tech Admissions 2024

Apply

Asia's Only University with the Highest US & UK Accreditation

ITM SLS Baroda University B.Tech Admissions 2024

Apply

Approved by UGC, PCI, & COA. Best Innovative Private Institute in Gujarat. 600+ Recruiters, 3000+ Students Placed, 1500+ Industrial Internship Opportunities, 250+ International Internships.