Spherical Mirror Formula And Magnification - Practice Questions & MCQ

Mirror formula

Let the object distance (u), image distance (v) and focal length (f). 

The following sign convention is used for measuring various distances in the ray diagrams of spherical mirrors:

• All distances are measured from the pole of the mirror.

• Distances measured in the direction of the incident ray are positive and the distances measured in the direction opposite to that of the incident rays are negative.

• Distances measured above the principal axis are positive and that measured below the principal axis are negative.

$
\begin{aligned}
& \text { In } \triangle A B C \text { and } \triangle A^{\prime} B^{\prime} C^{\prime} \\
& \triangle A B C \sim \Delta A^{\prime} B^{\prime} C[\text { AA similarity }] \\
& \frac{A B}{A^{\prime} B^{\prime}}=\frac{A C}{A^{\prime} C^{\prime}} \ldots \text { (1) }
\end{aligned}
$

Similarly, In $\triangle F P E$ and $\triangle A^{\prime} B^{\prime} F^{\prime}$

$
\begin{aligned}
& \frac{E P}{A^{\prime} B^{\prime}}=\frac{P F}{A^{\prime} F} \\
& \frac{A B}{A^{\prime} B^{\prime}}=\frac{P F}{A^{\prime} F}[\mathrm{AB}=\mathrm{EP}]
\end{aligned}
$

From (i) \&(ii)

$
\begin{aligned}
& \frac{A C}{A^{\prime} C}=\frac{P F}{A^{\prime} F} \\
& =>\frac{A^{\prime} C}{A C}=\frac{A^{\prime} F}{P F} \\
& =>\frac{\left(C P-A^{\prime} P\right)}{(A P-C P)}=\frac{\left(A^{\prime} P-P F\right)}{P F}
\end{aligned}
$

Now, $^{P F}=-f ; C P=2 P F=-2 f ; A P=-u$ and $A^{\prime} P=-v$
Put these values in the above relation:

$
\begin{aligned}
& \Longrightarrow \frac{[(-2 f)-(-v)]}{(-u)-(-2 f)}=\frac{[(-v)-(-f)]}{(-f)} \\
& \Longrightarrow u v=f v+u f \\
& \Longrightarrow \frac{1}{f}=\frac{1}{u}+\frac{1}{v}
\end{aligned}
$
 

Magnification in Spherical mirrors: 

lateral magnification: 

The lateral magnification is defined as the ratio:

$
m_v=\frac{\text { height of image }}{\text { height of object }}=\frac{h_i}{h_0}
$

To compute the vertical magnification, consider the extended object OA shown in Figure. The base of the object, O will map
on to a point I on the principal axis which can be determined from the equation $\quad \frac{1}{u}+\frac{1}{v}=\frac{1}{f}$

The image of the top of the object A, will map on to a point A' that will lie on the perpendicular through I. The exact location can be determined by drawing a ray from A passing through the pole and intercepting the line through I at A'.

Consider the triangles APO and $\mathrm{A}^{\prime} \mathrm{PI}$ in the figure. As the two triangles are similar, we get,

$
\tan \alpha=\frac{A O}{P O}=\frac{A^{\prime} I}{P I} \quad \text { or } \quad \frac{A^{\prime} I}{A O}=\frac{P I}{P O}
$

Applying the sign convention, we get, $\mathrm{u}=-\mathrm{PO}$

$
v=-P I \Rightarrow h_0=+A O \Rightarrow h_i=-A^{\prime} I
$

Therefore, $-\frac{h_i}{h_0}=\frac{v}{u} \quad$ or $\quad m_v=\frac{h_i}{h_0}=-\frac{v}{u}$
magnification formula can be modified as:

$
m=\frac{-v}{u}=\frac{f}{f-u}=\frac{f-v}{f}
$

Longitudinal magnification: When object lies along the principal axis then its axial magnification ' $m$ ' is given by

$
m=\frac{I}{O}=\frac{-\left(v_2-v_1\right)}{\left(u_2-u_1\right)}
$

If the object is small,

$
m=-\frac{d v}{d u}=\left(\frac{v}{u}\right)^2=\left(\frac{f}{f-u}\right)^2=\left(\frac{f-v}{f}\right)^2
$
 

Relation between the velocity of object and mirror in Spherical mirror

Case I: when the object moves along the principal axis

When we differentiate equation $\frac{1}{v}+\frac{1}{u}=\frac{1}{v}$ with respect to time.

$
\begin{aligned}
& \Rightarrow \quad-\frac{1}{v^2} \frac{d v}{d t}-\frac{1}{u^2} \frac{d u}{d t}=0 \\
& \Rightarrow \quad-\frac{1}{v^2} V_{i m}-\frac{1}{u^2} V_{O M}=0 \\
& \\
& \quad \frac{d v}{d t}=v_{i m}=\text { velocity of image w.r.t. miror } \\
& \Rightarrow \quad V_{i m}=-\frac{v^2}{u^2} V_{O M} \\
& \frac{d u}{d t}=v_{o M}=\text { velocity of object w.r.t. mirror } \\
& V_{i m}=-m^2 V_{O M} \\
& \Longrightarrow V_i-V_m=-m^2\left(V_O-V_m\right)
\end{aligned}
$

Therefore, $\Longrightarrow V_i=-m^2 V_O$ when the mirror is at rest along the principal axis.

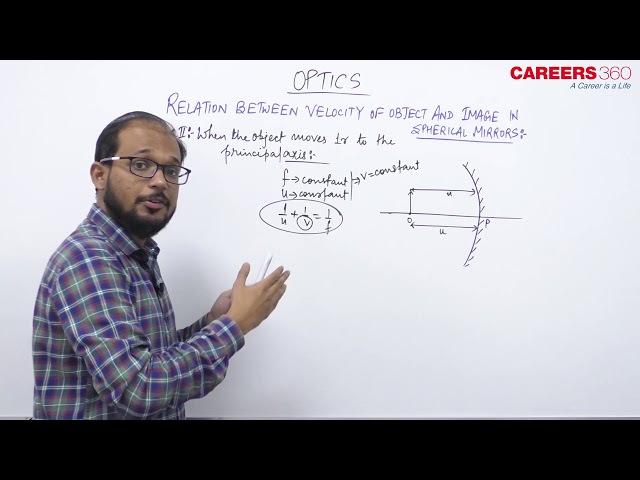
Case II: when the object moves perpendicular to the principal axis

When an object is moving perpendicular to the principal axis. This time  (image distance) and  (object distance) are constant. 

Therefore we have the following relation:

$
\frac{h_i}{h_o}=\frac{v}{u} \text { or } h_2=\left(\frac{-v}{u}\right) h_1
$

Also, the $x$-coordinates of both image and object remain constant. On differentiating the above relation w.r.t time we get,

$
\frac{d h_i}{d t}=-\frac{v}{u} \frac{d h_o}{d t}
$

Here, $\frac{d h_o}{d t}$ denotes the velocity of object perpendicular to the principal axis and $\frac{d h_i}{d t}$ denotes velocity of the image perpendicular to the principal axis.

Hence we can conclude that,

$
\begin{aligned}
& \frac{d h_i}{d t}=-\frac{v}{u} \frac{d h_o}{d t} \\
& \Longrightarrow\left(V_{i m}\right)_y=\frac{-v}{u}\left(V_{o m}\right)_y \\
& \Longrightarrow\left(V_{i m}\right)=m\left(V_{o m}\right)_y
\end{aligned}
$
 

Newton's Formula:

As we know that the mirror formula is given as 

$\frac{1}{v}+\frac{1}{u}=\frac{1}{f}$

Let's assume, $x=$ distance of the object from focus

$$
y=\text { distance of the image from focus }
$$

Newton's formula is useful for calculating the image position for a curved mirror.
The diagram shows the position of an object and its image formed by a concave mirror.

Let the distances of the object and image from the principal focus of the mirror be x and y respectively.

Then: Object distance $(u)=f+x$ and Image distance $(v)=f+y$
Using the mirror formula $\frac{1}{v}+\frac{1}{u}=\frac{1}{f}$ we have: $\frac{1}{f+x}+\frac{1}{f+y}=\frac{1}{f}$
and simplifying this we get: $f^2=x y$