Refraction Of Light Through Glass Slab - Practice Questions & MCQ

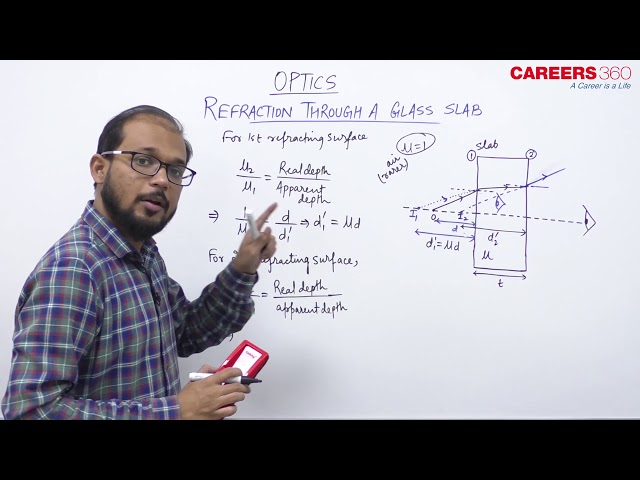
Consider an object O placed at distance d in front of a glass slab of thickness "t" and refractive index $\mu$. The observer is on
the other side of the slab. A ray of light from the object first refracts at the surface 1 and then refracts at the surface 2 before reaching the observer as shown in the above figure.

So for the refraction at the surface (1)
Apparent depth, $d_1^{\prime}=\frac{d_{\text {real }}}{n_{\text {relative }}}=\frac{d}{\left(\frac{n_{\text {incident }}}{n_{\text {reftaction }}}\right)}=\frac{d}{\frac{1}{\mu}}=d \mu$

Similarly for the refraction at the surface (2)
Apparent depth, $d_2^{\prime}=\frac{d_{\text {real }}}{n_{\text {relative }}}=\frac{d_1^{\prime}+t}{\left(\frac{n_{\text {incident }}}{n_{\text {reftaction }}}\right)}=\frac{d_1^{\prime}+t}{\frac{\mu}{1}}=\frac{d_1 \mu+t}{\mu}$

As you observe, The refracting surfaces of a glass slab are parallel to each other. When a light ray passes through a glass slab it is refracted twice at the two parallel faces and finally emerges out parallel to its incident direction.
i.e. the ray undergoes no deviation $(\delta=0$ ).
the object appears to be shifted towards the slab by the distance known as apparent shift or Normal shift.
And the apparent shift= $\mathrm{OA}-\mathrm{I}_2 \mathrm{~A}$
I.e Apparant shift $=t\left\{1-\frac{1}{\mu}\right\}$

If the slab is placed in the medium of the refractive index $\mu_{\text {sur }}$
then Apparant shift $=t\left\{1-\frac{\mu_{o u r}}{\mu}\right\}$

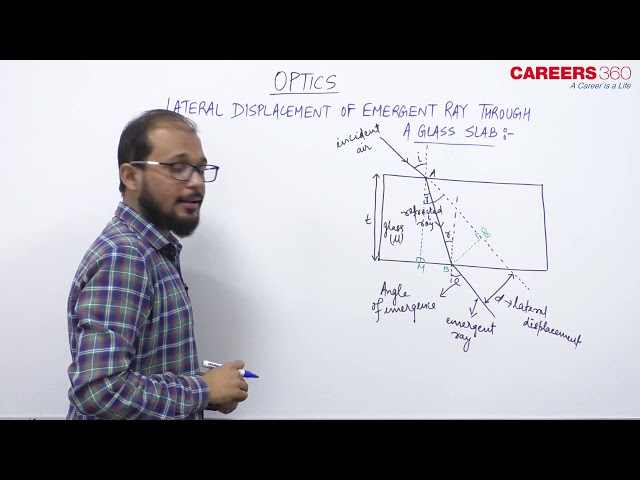
In the above figure Incident, ray AO is an incident on the EF surface of the slab at an angle of incident i, and PB is the emergent ray emerging out of the HG surface of the slab.

for the surface EF

Applying Snell's law at the surface EF and HG
$\mu_a \sin i=\mu \sin r \quad$ and $\quad \mu \sin r^{\prime}=\mu_a \sin e$
$U \operatorname{sing} \quad r^{\prime}=r$ and $\mu_a=1$, we get $\sin i=\sin e$ or $e=i$
i.e the emergent ray is parallel to the incident ray.

If PQ is the perpendicular dropped from P on the incident ray produced.
Then $P Q=d$ is known as lateral displacement which is given as

$
d=P Q=O P \sin (i-r)=\frac{O M}{\cos r} \sin (i-r)=\frac{t \sin (i-r)}{\cos r}
$

If $i$ is very small, $r$ is also very small, then $\quad d=\left(1-\frac{1}{\mu}\right) t i$