Rolling Without Slipping - Practice Questions & MCQ

 

• The linear velocity of different points 

In pure Translation-

In pure Rotation-

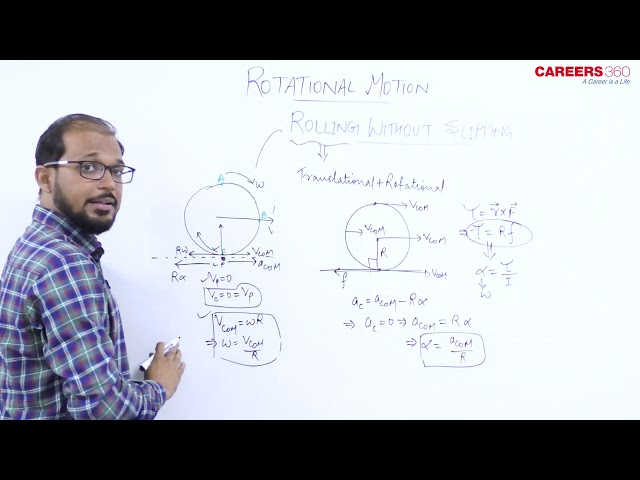
And in Rolling all points of a rigid body have same angular speed$(\omega)$ but different linear speed.

      I.e

• - During Rolling motion
  ${ }_{\text {If }} V_{c m}>R w \rightarrow$ slipping motion
  ${ }_{\text {If }} V_{c m}=R w \rightarrow$ pure rolling
  If $V_{c m}<R w \rightarrow$ skidding motion

 

When the object rolls across a surface such that there is no relative motion of object and surface at the point of contact, the motion is called rolling without slipping.

Here the point of contact is P.

Friction force is available between object and surface but work done by it is zero because there is no relative motion between body and surface at the point of contact.

Or we can say No dissipation of energy is there due to friction.

I.e., Energy is conserved.

Which is

$
K_{n e t}=K_T+K_R=\frac{1}{2} m V^2+\frac{1}{2} I \omega^2
$

Now using $V=\omega \cdot R$

And using

$
K_{n e t}=\frac{1}{2} m V^2+\frac{1}{2} I \omega^2=\frac{1}{2}\left(I+m R^2\right) \omega^2
$

Where I = moment of inertia of the rolling body about its centre 'O'
And using Parallel axis theorem
We can write $I_p=I+m R^2$

So we can write

$
K_{n e t}=\frac{1}{2} I_p \omega^2
$
 

 

Where $I_{p}=\text { moment of inertia of rolling body about point of contact }  \mathrm{P}$.

So this Rolling motion of a body is equivalent to a pure rotation about an axis passing through the point of contact (here through P ) with the same angular velocity $\omega$.
Here axis passing through the point of contact $P$ is also known as Instantaneous axis of rotation.
(Instantaneous axis of rotation-Motion of an object may look as pure rotation about a point that has zero velocity.)
- Net Kinetic Energy for different rolling bodies

$
\mathrm{As~} K_{n e t}=K_T+K_R=\frac{1}{2} m V^2\left(1+\frac{K^2}{R^2}\right)
$

$\frac{K^2}{R^2}$ will have different values for different bodies.

 

Rolling body	$\frac{K^2}{R^2}$	$K_{n e t}$
Ring Or Cylindrical shell	$1$	$m V^2$
Disc Or solid cylinder	$\frac{1}{2}$	$\frac{3}{4} m V^2$
Solid sphere	$\frac{2}{5}$	$\frac{7}{10} m V^2$
Hollow sphere	$\frac{2}{3}$	$\frac{5}{6} m V^2$

 

• - The direction of friction-

  Kinetic friction will always oppose the rolling motion. While Static friction on the other hand only opposes the tendency of an object to move.
  1. When an external force is in the upward diametric part
  - If $K^2=R x$ then no friction will act
  - If $K^2>R x$ then Friction will act in the backward direction
  - If $K^2<R x$ then Friction will act in a forward direction
  2. If an external force is in the lower diametric part,

  Then friction always act backwards