Centre Of Mass Of A Triangle - Practice Questions & MCQ

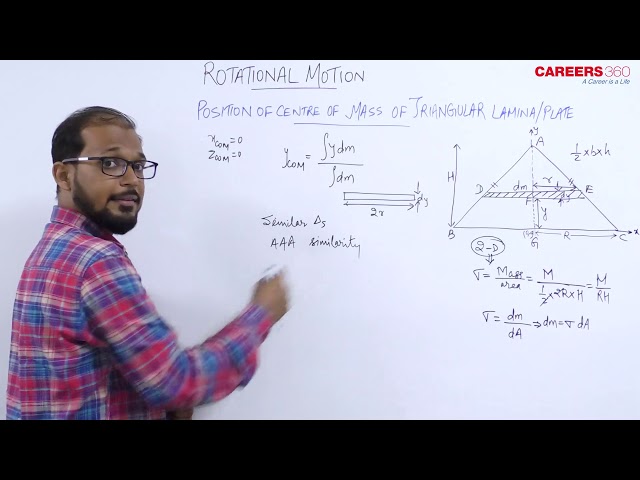
Have a look at the figure of A triangular plate as shown in figure.

Since it is symmetrical about $y$-axis on both sides of the origin
So we can say that its $x_{c m}=0$
And its $z_{c m}=0$ as z-coordinate is zero for all particles of semicircular ring.
Now we will calculate its $y_{c m}$ which is given by

$
y_{c m}=\frac{\int y \cdot d m}{\int d m}
$

For this take an elemental strip of mass dm and thickness dy at distance y from origin on y -axis
As shown in figure

$\triangle A D E$ and $\triangle A B C$ will be similar

So,

$
\begin{aligned}
\frac{r}{R} & =\frac{H-y}{H} \\
r & =\left(\frac{H-y}{H}\right) R \\
\sigma & =\frac{\text { mass }}{\text { area }}=\frac{M}{\frac{1}{2} *(2 R) * H} \\
\sigma & =\frac{M}{R H}
\end{aligned}
$

And,

$
\begin{aligned}
& \text { And, } \quad d m=\sigma d A=\sigma(2 r d y) \\
& y_{c m}=\frac{\int y \sigma d A}{M} \\
& y_{c m}=\frac{\int_H^0 y \cdot \sigma d y \cdot 2\left(\frac{H-y}{H}\right) \cdot R}{M}=\frac{H}{3} \\
& \text { So, } \quad \mathbf{y}_{\mathbf{c m}}=\frac{\mathbf{H}}{\mathbf{3}} \\
& \text { from base }
\end{aligned}
$