JEE Main Registration 2025 Session 1 (Open) - Link, Last Date, Fees, How to Apply

Centre Of Mass Of Hollow Cone - Practice Questions & MCQ

Edited By admin | Updated on Sep 18, 2023 18:34 AM | #JEE Main

Quick Facts

4 Questions around this concept.

Solve by difficulty

A hollow cone and a hollow semicircular shell are placed as shown in the diagram. Each has mass M . What is the y-coordinate of COM of system

A

$\frac{10}{13}R$

B

$\frac{5}{7}R$

C

$\frac{3}{7}R$

D

$\frac{13}{12}R$

Concepts Covered - 1

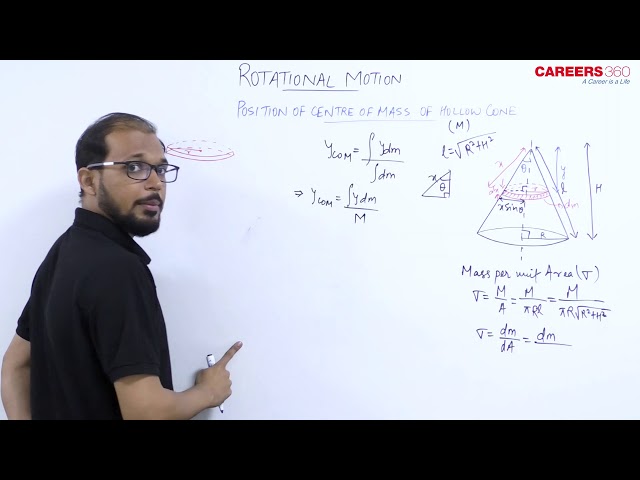
Position of centre of mass for Hollow Cone

Have a look at the figure of Hollow Cone

Since it is symmetrical about y-axis

So we can say that its $x_{cm} = 0$ and $z_{cm} = 0$

Now we will calculate its ${y_{cm}$ which is given by

$y_{cm} = \frac{\int y.dm}{\int dm}$

So Take a small elemental ring of mass dm of radius r at a vertical distance y from O as shown in figure.

And $r=xsin\theta , \ and \ y=xcos\theta$

Since our element mass is ring so its C.O.M will lie on the y-axis.

Now $dm=\sigma dA=\sigma (2\pi xsin\theta )dx$

Where $\sigma = \frac{M}{\pi R*\sqrt{R^2+H^2}}$

So $dm=\frac{2Mxdx}{R^2+H^2}$

$y_{cm}=\frac{1}{M} \int ydm=\frac{1}{M}\int_{0}^{\sqrt{R^2+H^2}}xcos\theta *\frac{2Mxdx}{R^2+H^2}=\frac{2H}{3}$

So $\mathbf{y_{cm}= \frac{2H}{3}}$ from O.

Study it with Videos

Position of centre of mass for Hollow Cone

Study other Related Concepts

Centre Of Mass Of Hollow Cone Current Topic

Rotational Motion Of Rigid Body

Center Of Mass Of The Uniform Rod

Centre Of Mass Of Semicircular Ring

Centre Of Mass Of Semicircular Disc

Centre Of Mass Of A Triangle

Centre Of Mass Of Hollow Hemisphere

Centre Of Mass Of Solid Hemisphere

Centre Of Mass Of Hollow Cone

Centre Of Mass Of A Solid Cone

"Stay in the loop. Receive exam news, study resources, and expert advice!"

Get Answer to all your questions

JEE Main Articles

JEE Main Important Formulas 2025 for Physics, Chemistry, Maths

Nov 21, 2024

Scholarship for BTech Students in India 2025 - Eligibility, Amount, How to Apply

Nov 21, 2024

JEE Main Registration 2025 Last Date Tomorrow, Direct Link at jeemain.nta.nic.in, Live Updates

Nov 21, 2024

JEE Main 2025 Exam Centres in Andhra Pradesh (AP)

Nov 21, 2024

Upcoming Engineering Exams 2025 - Latest Updates on JEE, VITEEE, BITSAT, MHT CET, State Exams

Nov 21, 2024

Engineering Entrance Exams Application Date 2025 & Details

Nov 21, 2024

MNNIT Allahabad Cutoff JEE Main 2024 - Check Previous Year Cutoff Here

Nov 21, 2024

NIT Calicut Cutoff JEE Main 2024 - Opening and Closing Rank

Nov 21, 2024

B.Tech/B.Arch Admissions OPEN

UPES B.Tech Admissions 2025

Ranked #42 among Engineering colleges in India by NIRF | Highest CTC 50 LPA , 100% Placements

Amrita University B.Tech 2025

Recognized as Institute of Eminence by Govt. of India | NAAC ‘A++’ Grade | Upto 75% Scholarships

Dayananda Sagar University B.Tech Admissions 2025

60+ Years of Education Legacy | UGC & AICTE Approved | Prestigious Scholarship Worth 6 Crores | H-CTC 35 LPA

Parul University B.Tech Admissions 2025

India's youngest NAAC A++ accredited University | NIRF rank band 151-200 | 2200 Recruiters | 45.98 Lakhs Highest Package

Lovely Professional University B.Tech Admissions 2025

India's Largest University | NAAC A++ Accredited | 100% Placement Record | Highest Package Offered : 3 Cr PA

Atlas SkillTech University | B.Tech Admissions

Hands on Mentoring and Code Coaching | Cutting Edge Curriculum with Real World Application

View All Application Forms

News and Notifications

9 hours ago :

Check JEE Main 2025 registration live updates here.

November 05, 2024 - 04:02 PM IST :

NTA has announced the JEE Main exam date 2025 for sessions 1 and 2.

BTech Admission 2025 Live: JEE Mains registrations closing tomorrow; VITEEE, MET exam dates out

November 21, 2024 - 03:28 PM IST

JEE Main 2025 registration ends tomorrow; changes introduced in exam this year

November 21, 2024 - 08:34 AM IST

JEE Main 2025 Live: NTA to close JEE registration on Friday; apply at jeemain.nta.nic.in, correction window

November 20, 2024 - 09:45 PM IST

BTech Exams 2025: JEE Main application correction dates out; MHT CET syllabus, VITEEE exam date

November 19, 2024 - 10:05 PM IST

JEE Mains 2025 session 1 registration closing soon; application correction window open till November 27

November 19, 2024 - 03:45 PM IST

JEE Main 2025 exam date will not be changed in case of clash with other exams; NTA answers FAQs

November 19, 2024 - 07:59 AM IST

JEE Mains 2025: Application correction facility from November 26 to 27; NTA answers FAQs

November 18, 2024 - 10:52 PM IST

Download Careers360 App

All this at the convenience of your phone

Regular Exam Updates
Best College Recommendations
College & Rank predictors
Detailed Books and Sample Papers
Question and Answers

Scan and download the app

OR

Joint Entrance Examination (Main)

JEE Main 2025 Sample Papers