Mayer's Formula - Practice Questions & MCQ

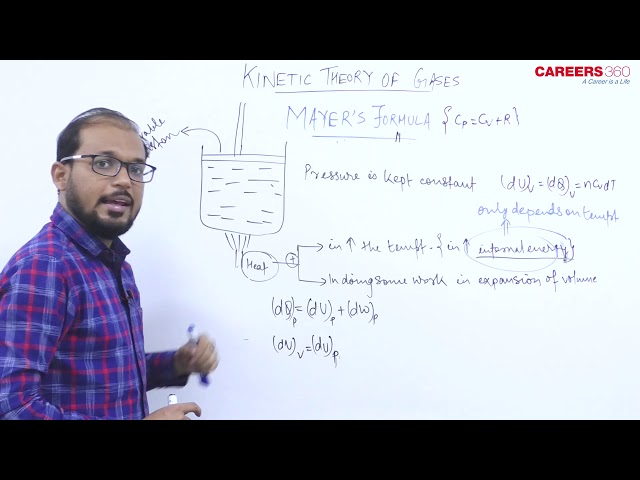
• - Mayer's formula- As we know

  Molar Specific heat of the gas at constant volume $=C_v$
  and Molar Specific heat capacity at constant pressure $=C_p$
  Mayer's formula gives the relation between $C_p$ and $C_v$ as $C_p=C_v+R$
  or we can say that molar Mayer's formula shows that specific heat at constant pressure is greater than that at constant volume.
  - Specific Heat in Terms of Degree of Freedom
  1.Molar Specific heat of the gas at constant volume $\left(C_v\right)$

  For a gas at temperature $T$, the internal energy
  $U=\frac{f}{2} n R T \Rightarrow$ Change in energy $\Delta U=\frac{f}{2} n R \Delta T \ldots$
  Also, as we know for any gas heat supplied at constant volume

  $
  (\Delta Q)_V=n C_V \Delta T=\Delta U \ldots \ldots(i i)
  $

  
  From the equation (i) and (ii)

  $
  C_v=\frac{f R}{2}
  $
   

where 

f = degree of freedom

R= Universal gas constant

2. Molar Specific heat of the gas at constant pressure ( $C_p$ )

From Mayer's formula, we know that $C_p=C_v+R$

$
\Rightarrow C_P=C_V+R=\frac{f}{2} R+R=\left(\frac{f}{2}+1\right) R
$

3. Atomicity or adiabatic coefficient ( ${ }^{\prime}$ )

It is the ratio of $C_p$ to $C_v$

$
\gamma=\frac{C_p}{C_v}=1+\frac{2}{f}
$

Value of $\gamma$ is always more than 1
for Monoatomic gas

$
\gamma=\frac{5}{3}
$

for Diatomic gas

$
\gamma=\frac{7}{5}
$

for Triatomic gas

$
\gamma=\frac{4}{3}
$
 

•  Gaseous Mixture

If two non-reactive gases A and B are enclosed in a vessel of volume V .
In the mixture $\mathrm{n}_1$ mole of Gas A (having Specific capacities as $C_{p 1}$ and $C_{v 1}$, Degree of freedom $f_1$ and Molar mass as $M_1$ ) is mixed with $\mathrm{n}_2$ mole of Gas B (having Specific capacities as $C_{p 2}$ and $C_{v 2}$, Degree of freedom $f_2$ and Molar mass as $M_2$ )

Then Specific heat of the mixture at constant volume will be

$
C_{v_{m i x}}=\frac{n_1 C_{v_1}+n_2 C_{v_2}}{n_1+n_2}
$

Similarly, Specific heat of the mixture at constant pressure will be

$
C_{p_{m i x}}=\frac{n_1 C_{p_1}+n_2 C_{p_2}}{n_1+n_2}
$

And adiabatic coefficient $(\gamma)$ of the mixture is given by

$
\gamma_{\text {mixure }}=\frac{C_{p_{m i x}}}{C_{v_{m x}}}=\frac{\frac{\left(n_1 C_{p_1}+n_2 C_{p_2}\right)}{n_1+n_2}}{\frac{\left(n_1 C_{v_1}+n_2 C_{v_2}\right)}{n_1+n_2}}=\frac{\left(n_1 C_{p_1}+n_2 C_{p_2}\right)}{\left(n_1 C_{v_1}+n_2 C_{v_2}\right)}
$

Also

$
\frac{1}{\gamma_{\operatorname{mix}}-1}=\frac{\frac{n_1}{\gamma_1-1}+\frac{n_2}{\gamma_2-1}}{n_1+n_2}
$

Similarly, the Degree of freedom of mixture is given as

$
f_{\operatorname{mix}}=\frac{n_1 f_1+n_2 f_2}{n_1+n_2}
$

Similarly, the molar mass of the mixture

$
M_{m i x}=\frac{n_1 M_1+n_2 M_2}{n_1+n_2}
$