UPES B.Tech Admissions 2026
Ranked #43 among Engineering colleges in India by NIRF | Highest Package 1.3 CR , 100% Placements
Ideal gas equation is considered one of the most asked concept.
78 Questions around this concept.
Which of the following shows the correct relationship between the pressure ‘P’ and density ρ of an ideal gas at constant temperature?
‘n’ moles of an ideal gas undergoes a process A→B as shown in the figure. The maximum temperature of the gas during the process will be :

Which of the following graphs represent the behavior of an ideal gas? Symbols have their usual meaning.
JEE Main 2026: Preparation Tips & Study Plan | Previous 10 Year Questions
JEE Main 2026: 100 Days Study Plan | High Scoring Chapters and Topics | Preparation Tips
JEE Main 2026: Top 30 Most Repeated Questions | Chapter Wise PYQs | Mock Test
JEE Main 2025 Most Scoring Concept: January Session | April Session
For the P-V diagram given for an ideal gas,

out of the following which one correctly represents the T-P diagram ?
Which of the following diagrams (figure) depicts ideal gas behaviour?
One mole of an ideal gas undergoes a linear process as shown in the figure below. Its temperature expressed as a function of volume V is,
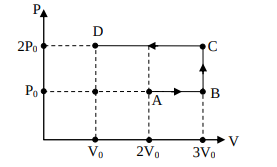
Using the given P-V diagram, the work done by an ideal gas along the path ABCD is-
Ranked #43 among Engineering colleges in India by NIRF | Highest Package 1.3 CR , 100% Placements
Campuses in Ropar, Agartala, Aizawl, Ajmer, Aurangabad, Calicut, Imphal, Itanagar, Kohima, Gorakhpur, Patna & Srinagar
The equation which relates the pressure (P), volume (V) and temperature (T) of the given state of an ideal gas is known as an ideal gas equation or equation of state.
From Boyle's law, we get
$
V \propto \frac{1}{P}
$
and From Charle's Law, we get $V \propto T$.
And from Avogadro's Law, we get $V \propto n$
And from equation (1), (2), (3)
we can write
$
\begin{aligned}
& V \propto \frac{n T}{P} \\
& \text { or } \frac{P V}{n T}=\text { constant } \\
& \text { or } \frac{P V}{n T}=R(\text { where } R \text { is proportionality constant }) \\
& \text { or } P V=n R T
\end{aligned}
$
So Ideal Gas Equation is given as
$
P V=n R T
$
where
T= Temperature
P= pressure of ideal gas
V= volume
n= numbers of mole
R = universal gas constant
At S.T.P. the value of the universal gas constant is the same for all gases.
And its value is given as
$
R=8.31 \frac{\text { Joule }}{\text { mole } \times \text { Kelvin }}=2 \frac{\mathrm{cal}}{\text { mole } \times \text { Kelvin }}
$
And its Dimension is : $\left[M L^2 T^{-2} \theta^{-1}\right]$
- Boltzman's constant (k)-
It is represented by per mole gas constant.
$
\text { i.e } k=\frac{R}{N}=\frac{8.31}{6.023 \times 10^{23}}=1.38 \times 10^{-23} \mathrm{~J} / \mathrm{K}
$
- Specific gas constant (r)-
It is represented by per gram gas constant.
i.e. $\quad r=\frac{R}{M}$
It's unit is $\frac{\text { Joule }}{\mathrm{gm} \times \text { kelvin }}$
"Stay in the loop. Receive exam news, study resources, and expert advice!"
