Degree Of Freedom - Practice Questions & MCQ

Degree of freedom-

The degree of freedom of systems is defined as the possible independent motions, systems can have.

Or 

The degree of freedom of systems is defined as the number of independent coordinates required to describe the system completely.

The independent motions can be translational, rotational or vibrational or any combination of these.

So the degree of freedom is of three types :
(i) Translational degree of freedom
(ii) Rotational degree of freedom
(iii) Vibrational degree of freedom

The degree of freedom is denoted by .

And it is given by

$
f=3 N-R
$

Where
$N=$ no. of particle
$R=$ no. of relation
- Value of degree of freedom for
1.Monoatomic gas-

A monoatomic gas can only have a translational degree of freedom.
l.e $f=3$

Example- $\mathrm{He}, \mathrm{Ne}, \mathrm{Ar}$
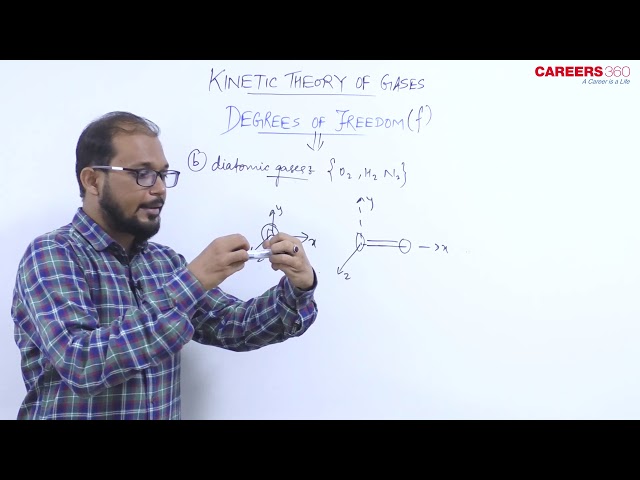
2. Diatomic gas

A diatomic gas can have three translational degrees of freedom and two rotational degrees of freedom.
I.e $f=5$

Example- $\mathrm{H}_2, \mathrm{O}_2, \mathrm{~N}_2$
3. Triatomic gas

A triatomic gas can have three translational degrees of freedom and three rotational degrees of freedom.
I.e $f=6$

Example- $\mathrm{H}_2 \mathrm{O}$

• Note-

The above degrees of freedom are shown at room temperature. Further at high temperature, in the case of diatomic or polyatomic molecules,
the atoms within the molecule may also vibrate with respect to each other. In such cases, the molecule will have 2 additional degrees of freedom, due to vibrational motion. I.e One for the potential energy and one for the kinetic energy of vibration.

So A diatomic molecule that is free to vibrate (in addition to translation and rotation) will have 7 degrees of freedom.