Energy Stored In An Inductor - Practice Questions & MCQ

Energy stored in an inductor (U)-

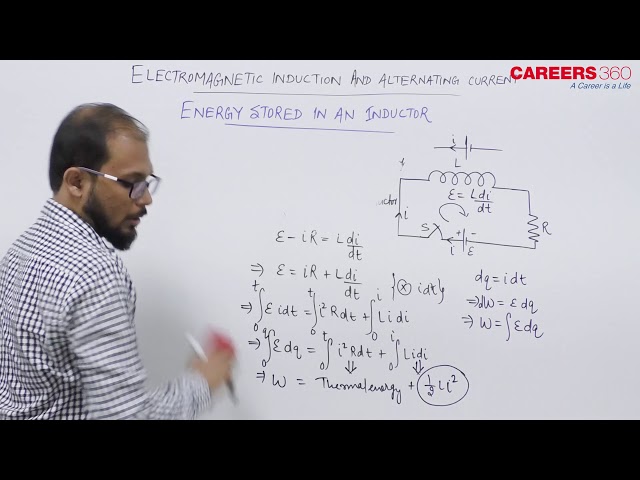
In building a steady current in the circuit, the source emf has to do work against of self-inductance of the coil and whatever energy
consumed for this work stored in the magnetic field of coil this energy called as magnetic potential energy (U) of the coil.

When an electric current i is flowing in an inductor, there is energy stored in the magnetic field. Considering a pure inductor L, the instantaneous power which must be supplied to initiate the current in the inductor is

$
P=i v=L i \frac{d i}{d t}
$

The work done by the voltage source during a time interval $d t$ is

$
d W=P d t=i L \frac{d i}{d t} d t=\text { Lidi }
$

total work $W$ done in establishing the final current $I$ in the inductor

$
\mathrm{W}=\int_0^t P d t=\int_0^I L i d i=\frac{1}{2} L I^2
$

So Energy stored in the magnetic field of the inductor is given as

$
U=\frac{1}{2} L I^2
$

The energy density (u)/Energy per unit volumeusing $U=\frac{1}{2} L I^2$

for the solenoid field, we can write 

\begin{aligned}
& U=\frac{1}{2}(L i) i=\frac{N \phi i}{2} \\
& u=\frac{U}{V}=\frac{B^2}{2 \mu_0}
\end{aligned}