Relation Between Object And Image Velocity In Lens - Practice Questions & MCQ

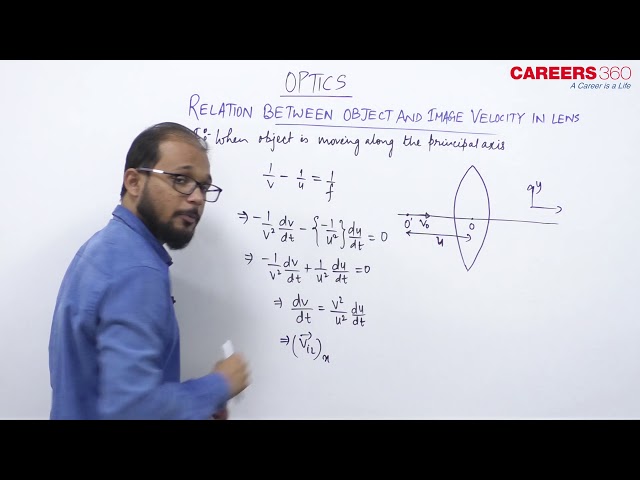
Relation between object and image velocity in lens - 

Case 1 : When object is moving along the principal axis -

As we have learned the following equation -

$
\frac{1}{v}-\frac{1}{u}=\frac{1}{f}
$

After differentiation -

$
\begin{aligned}
& \Rightarrow \frac{-1}{v^2} \frac{d v}{d t}-\left\{\frac{-1}{u^2}\right\} \frac{d u}{d t}=0 \\
& \Rightarrow \frac{-1}{v^2} \frac{d v}{d t}+\frac{1}{u^2} \frac{d u}{d t}=0 \\
& \Rightarrow \frac{d v}{d t}=\frac{v^2}{u^2} \frac{d u}{d t} \\
& \Rightarrow\left(\vec{v}_{i L}\right)_x=\frac{v^2}{u^2}\left(\vec{v}_{o L}\right)_x \\
& \Rightarrow\left(\vec{v}_{i L}\right)_x=m^2\left(\vec{v}_{o L}\right)_x
\end{aligned}
$
 

Case 2 : When object is moving perpendicular to the principal axis -

$
\begin{aligned}
& m=\frac{h_i}{h_0}=\frac{v}{u} \\
& \Rightarrow u h_i=v h_o
\end{aligned}
$

After differntiation -

$
\begin{gathered}
\Rightarrow u \frac{d h_i}{d t}=v \frac{d h_0}{d t} \\
\Rightarrow \frac{d h_i}{d t}=\frac{v}{u} \frac{d h_0}{d t} \Rightarrow\left(\vec{V}_{i L}\right)_y=m\left(\vec{V}_{o L}\right)_y
\end{gathered}
$

Here, $\mathrm{m}=$ magnification,
$v=$ Position of image
$\mathrm{u}=$ Position of object
$\vec{V}=$ Velocity vector