Pair of Straight Line - Practice Questions & MCQ

Joint Equation of a Pair of Straight Lines

If the equation of two straight lines are $a_1 x+b_1 y+c_1=0$ and $a_2 x+b_2 y+c_2=0$, then combined or joint equation of these two lines is

$
\left(a_1 x+b_1 y+c_1\right)\left(a_2 x+b_2 y+c_2\right)=0
$
Multiplying the brackets we get

$
\Rightarrow a_1 a_2 x^2+\left(a_1 b_2+a_2 b_1\right) x y+b_1 b_2 y^2+\left(a_1 c_2+c_1 a_2\right) x+\left(b_1 c_2+c_1 b_2\right) y+c_1 c_2=0
$
Here the coefficients can be re-named and this equation can be re-written as

$
a x^2+2 h x y+b y^2+2 g x+2 f y+c=0
$
So, a pair of straight lines is represented by a two degree equation in x and y .

Condition for a general two degree equation to represent a pair of straight lines

A general two degree equation $\mathrm{ax}^2+2 \mathrm{hxy}+\mathrm{by} 2+2 \mathrm{gx}+2 \mathrm{fy}+\mathrm{c}=0$ may represent a
Pair of straight lines, or
Circle, or
Parabola, or
Ellipse, or
Hyperbola

To identify which of the following curves is represented by a given equation, we have a determinant

$
\Delta=\left|\begin{array}{lll}
a & h & g \\
h & b & f \\
g & f & c
\end{array}\right|
$
If this determinant equals zero, then the given equation represents a pair of straight lines. (The conditions for other curves will be seen in subsequent chapters).
Proof:
If we write the equation as a quadratic in $x$, where $a \neq 0$

$
a x^2+2 x(h y+g)+\left(b y^2+2 f y+c\right)=0
$
Solving for ' $x$ ', we get

$
\begin{aligned}
x & =\frac{-2(h y+g) \pm \sqrt{4(h y+g)^2-4 a\left(b y^2+2 f y+c\right)}}{2 a} \\
& =\frac{-(h y+g) \pm \sqrt{\left(h^2-a b\right) y^2+2(g h-a f) y+\left(g^2-a c\right)}}{a}
\end{aligned}
$

For this equation to represent two straight lines, we must have ‘x’ as a linear expression in ‘y’. For that, the value under the square root must be a perfect square of some linear expression in ‘y’. For this to happen, the Discriminant of the quadratic equation in y (the equation inside the root) must be zero.

Discriminant of $\left(h^2-a b\right) y^2+2(g h-a f) y+\left(g^2-a c\right)$ is 0
So,

$
\begin{aligned}
& \Rightarrow \quad 4(\mathrm{gh}-\mathrm{af})^2-4\left(\mathrm{~h}^2-\mathrm{ab}\right)\left(\mathrm{g}^2-\mathrm{ac}\right)=0 \\
& \Rightarrow \quad \mathrm{abc}+2 \mathrm{fgh}-\mathrm{af}^2-\mathrm{bg}^2-\mathrm{ch}^2=0
\end{aligned}
$
This is the condition for which $a x^2+2 h x y+b y 2+2 g x+2 f y+c=0$ represent a pair of straight line.
This condition can also be written in a determinant form as

$
\left|\begin{array}{lll}
a & h & g \\
h & b & f \\
g & f & c
\end{array}\right|=0
$
 

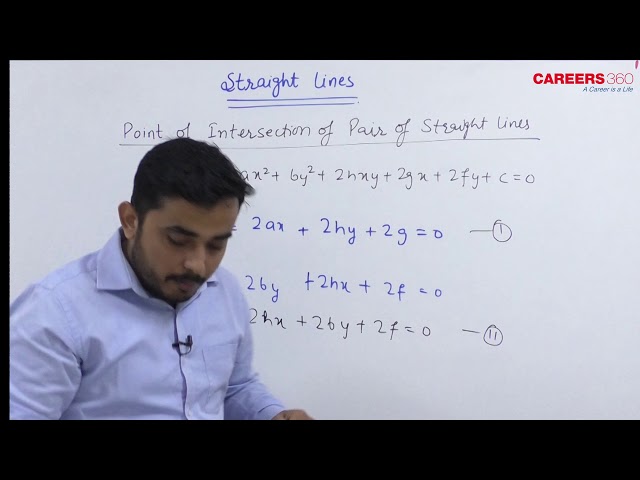
Point of Intersection of Pair of Straight Lines

To get the point of intersection, first find the separate equations of straight line and solve them simultaneously.

Or 

The point of intersection of pair of straight lines can also be determined with the help of partial differentiation
Let $\phi \equiv \mathrm{ax}^2+2 \mathrm{hxy}+\mathrm{by}^2+2 \mathrm{gx}+2 \mathrm{fy}+\mathrm{c}=0$
Differentiating $\phi$ with respect to $x$, keeping $y$ constant, we get,

$
\frac{\partial \phi}{\partial \mathrm{x}}=2 \mathrm{ax}+2 \mathrm{hy}+2 \mathrm{~g}
$
Similarly, differentiating $\phi$ with respect to $y$, keeping $x$ constant, we get

$
\frac{\partial \phi}{\partial \mathrm{y}}=2 \mathrm{hx}+2 \mathrm{by}+2 \mathrm{f}
$
For point of intersection, we get

$
\frac{\partial \phi}{\partial \mathrm{x}}=0 \text { and } \frac{\partial \phi}{\partial \mathrm{y}}=0
$
Thus, we have $a x+h y+g=0$ and $h x+b y+f=0$.
Solving the two equations, we get

$
\begin{aligned}
& \frac{x}{f h-b g}
\end{aligned}=\frac{y}{g h-a f}=\frac{1}{a b-h^2}, ~(x, y)=\left(\frac{b g-f h}{h^2-a b}, \frac{a f-g h}{h^2-a b}\right)
$
IMPORTANT NOTE
In the above equations, $2 a x+2 h y+2 g=0$ and $2 h x+2 b y+2 f=0$ are NOT the equations of components of straight line.