Moment Of Inertia Of Solid Cone - Practice Questions & MCQ

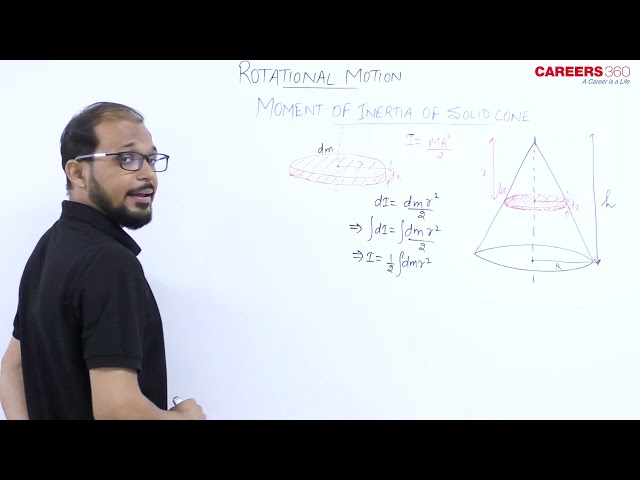
Let I=Moment of inertia of a solid cone about an axis through its C.O.M

To calculate I

Consider a solid cone of mass M, base radius R ,  and Height as h 

As shown in figure I is about the x axis and through its C.O.M

Now take an elemental disc of mass dm at a distance x from top as shown in figure

As The density of the cone is

$
\rho=\frac{M}{V}=\frac{M}{\frac{1}{3} \pi R^2 h}
$

So, $d m=\rho d V=\rho\left(\pi r^2 d x\right)$
Using similar triangle method we have

$
\frac{r}{x}=\frac{R}{h}
$

So, $x=\frac{r h}{R} \Rightarrow d x=\frac{h d r}{R}$
And for an elemental disc moment of inertia about x - axis is given by

$
d I=\frac{1}{2} * d m r^2
$
 

So,

$\begin{aligned} & d I=\frac{1}{2} * d m r^2 \\ & d I=\frac{1}{2} \rho \pi r^2 d x * r^2 \\ & d I=\frac{1}{2} \rho \pi r^2 * r^2 * \frac{h}{R} d r \\ & \int d I=\frac{1}{2} \rho \pi \frac{h}{R} \int r^4 d r \\ & \int d I=\frac{1}{2} * \frac{3 M}{\pi R^2 h} \pi \frac{h}{R} \int_0^R r^4 d r \\ & I=\frac{3}{2} * \frac{M}{R^3} * \frac{R^5}{5} \\ & \mathbf{I}=\frac{\mathbf{3}}{\mathbf{1 0}} * \mathbf{M R}^{\mathbf{2}}\end{aligned}$