Equation of The Plane Bisecting the Angle Between Two Planes - Practice Questions & MCQ

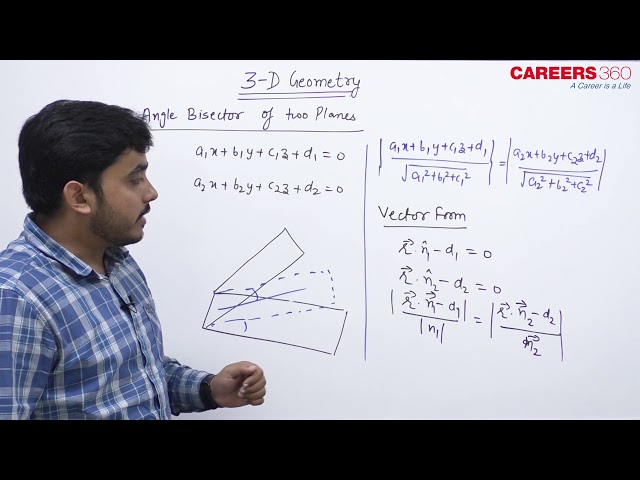
Cartesian Form

Equation of the planes bisecting the angle between the planes a₁x + b₁y + c₁z + d₁ = 0 and a₂x + b₂y + c₂z + d₂ = 0 is

$
\frac{a_1 x+b_1 y+c_1 z+d_1}{\sqrt{a_1^2+b_1^2+c_1^2}}= \pm \frac{a_2 x+b_2 y+c_2 z+d_2}{\sqrt{a_2^2+b_2^2+c_2^2}}
$

Proof:
Given planes are
$
\begin{array}{ll} 
& a_1 x+b_1 y+c_1 z+d_1=0 \\
\text { and } & a_2 x+b_2 y+c_2 z+d_2=0
\end{array}
$

Let P(x, y, z) be a point on the plane bisecting the angle between planes (i) and (ii).

Let PL and PM be the length of perpendiculars from P to planes (i) and (ii).

$\begin{array}{rlrl}\therefore & P L & =P M \\ \Rightarrow & & \left|\frac{a_1 x+b_1 y+c_1 z+d_1}{\sqrt{a_1^2+b_1^2+c_1^2}}\right| & =\left|\frac{a_2 x+b_2 y+c_2 z+d_2}{\sqrt{a_2^2+b_2^2+c_2^2}}\right| \\ \frac{a_1 x+b_1 y+c_1 z+d_1}{\sqrt{a_1^2+b_1^2+c_1^2}} & = \pm \frac{a_2 x+b_2 y+c_2 z+d_2}{\sqrt{a_2^2+b_2^2+c_2^2}}\end{array}$

This is an equation of planes bisecting the angles between the planes (i) and (ii).

Vector Form

Equation of the planes bisecting the angle between the planes $\overrightarrow{\mathbf{r}} \cdot \overrightarrow{\mathbf{n}}=d_1$ and $\overrightarrow{\mathbf{r}} \cdot \overrightarrow{\mathbf{n}}_2=d_2$ is
$
\left|\frac{\overrightarrow{\mathbf{r}} \cdot \overrightarrow{\mathbf{n}}_1-d_1}{\overrightarrow{\mathbf{n}}_1}\right|=\left|\frac{\overrightarrow{\mathbf{r}} \cdot \overrightarrow{\mathbf{n}}_2-d_2}{\overrightarrow{\mathbf{n}}_2}\right|
$

Bisector of the Angle between the Two Planes Containing the Origin
Let the equation of the two planes be
$
\begin{array}{ll} 
& a_1 x+b_1 y+c_1 z+d_1=0 \\
\text { and } & a_2 x+b_2 y+c_2 z+d_2=0
\end{array}
$
where $\mathrm{d}_1$ and $\mathrm{d}_2$ are positive.
Then the equation of the bisector of the angle between the planes (i) and (ii) containing the origin is
$
\frac{a_1 x+b_1 y+c_1 z+d_1}{\sqrt{a_1^2+b_1^2+c_1^2}}=\frac{a_2 x+b_2 y+c_2 z+d_2}{\sqrt{a_2^2+b_2^2+c_2^2}}
$

Bisector of the Acute and Obtuse Angle between Two Planes 

Let the equation of the two planes be
and
$
\begin{aligned}
& a_1 x+b_1 y+c_1 z+d_1=0 \\
& a_2 x+b_2 y+c_2 z+d_2=0
\end{aligned}
$
1. If $a_1 a_2+b_1 b_2+c_1 c_2>0$, then the equation of the bisector of the obtuse angle is,
$
\frac{\mathrm{a}_1 \mathrm{x}+\mathrm{b}_1 \mathrm{y}+\mathrm{c}_1 \mathrm{z}+\mathrm{d}_1}{\sqrt{\mathrm{a}_1^2+\mathrm{b}_1^2+\mathrm{c}_1^2}}=\frac{\mathrm{a}_2 \mathrm{x}+\mathrm{b}_2 \mathrm{y}+\mathrm{c}_2 \mathrm{z}+\mathrm{d}_2}{\sqrt{\mathrm{a}_2^2+\mathrm{b}_2^2+\mathrm{c}_2^2}}
$
2. If $a_1 a_2+b_1 b_2+c_1 c_2<0$, then the equation of the bisector of the obtuse angle is,
$
\frac{\mathrm{a}_1 \mathrm{x}+\mathrm{b}_1 \mathrm{y}+\mathrm{c}_1 \mathrm{z}+\mathrm{d}_1}{\sqrt{\mathrm{a}_1^2+\mathrm{b}_1^2+\mathrm{c}_1^2}}=-\frac{\mathrm{a}_2 \mathrm{x}+\mathrm{b}_2 \mathrm{y}+\mathrm{c}_2 \mathrm{z}+\mathrm{d}_2}{\sqrt{\mathrm{a}_2^2+\mathrm{b}_2^2+\mathrm{c}_2^2}}
$