MEDIUM
Amity University Noida B.Tech Admissions 2025
ApplyAmong Top 30 National Universities for Engineering (NIRF 2024) | 30+ Specializations | AI Powered Learning & State-of-the-Art Facilities
Degree of Dissociation - Practice Questions & MCQ
Edited By admin | Updated on Sep 18, 2023 18:35 AM | #JEE Main
Quick Facts
-
22 Questions around this concept.
Solve by difficulty
The equilibrium constants $K_{p_1}$ and $K_{p_2}$ for the reaction $X \rightleftharpoons 2 Y$ and $Z \rightleftharpoons P+Q$,respectively are in the ratio of $1: 9$. If degree of dissociation of $X$ and $Z$ be equal then the ratio of total pressures at these equilibria is
The degree of dissociation of PCl5 is 60%,then find out the observed molar mass of the mixture.
The equilibrium constants for the reactions $\mathrm{X}=2 \mathrm{Y}$ and $\mathrm{Z}=\mathrm{P}+\mathrm{Q}$ are $\mathrm{K}_1$ and $\mathrm{K}_2$, respectively. If the initial concentrations and the degree of dissociation of $X$ and $Z$ are the same, the ratio $K_1 / K_2$ is
New: JEE Main 2025 City Slip OUT; Download now | Preparation, Study Plan
JEE Main 2025: Sample Papers | Mock Tests | PYQs | High Scoring Topics | College Predictor
New: Meet Careers360 experts in your city and get guidance on shortlisting colleges
Apply to TOP B.Tech /BE Entrance exams: VITEEE | MET | AEEE | BITSAT
Consider the reaction
$\mathrm{X}_2 \mathrm{Y}(\mathrm{~g}) \rightleftharpoons \mathrm{X}_2(\mathrm{~g})+\frac{1}{2} \mathrm{Y}_2(\mathrm{~g})$
The equation representing correct relationship between the degree of dissociation ( x ) of $\mathrm{X}_2 \mathrm{Y}(\mathrm{g})$ with its equilibrium constant Kp is ______ .
Assume x to be very very small.
The degree of dissociation of $\mathrm{PCl}_5(\alpha)$ obeying the equilibrium, $\mathrm{PCl}_5(\mathrm{~g}) \leftrightarrow \mathrm{PCl}_3(\mathrm{~g})+$ Cl2(g) is approximately related to the pressure at equilibrium by
At equilibrium, the rate of dissolution of a solid solute in a volatile liquid solvent is __________.
Concepts Covered - 2
Degree of Dissociation
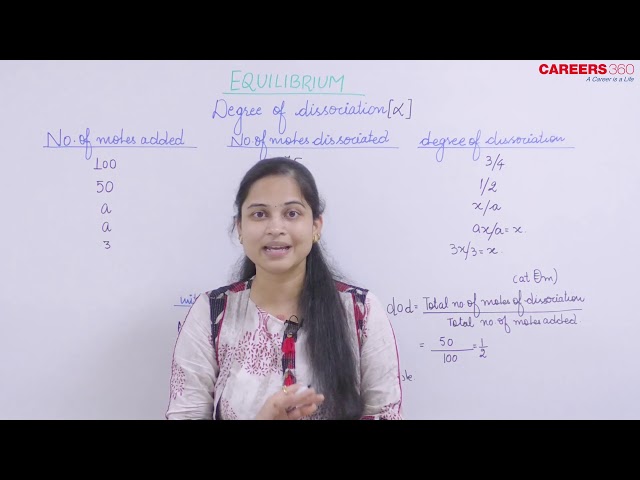
Degree of dissociation: It is the extent to which any reactant gets dissociated. It is denoted by .
If "a" be the initial number of moles and the number of moles dissociated be "x" then
Relation between degree of dissociation and Observed Molar mass/Vapor density
Due to dissociation, the total number of moles at equilibrium can be determined. Knowing the number of moles at equilibrium, the observed molar mass can be calculated.
Conversely, if the degree of dissociation is not known but the Observed Vapor density is available, then we can calculate the degree of dissociation.
We know about the law of conservation of mass
Using the above equation, we can calculate the unknown term required to be calculated.
Observed Density and Molar Mass
In equilibrium, the observed molar mass or average molar mass of the reactant is the total mass of the mixture divided by the total number of moles.
Initially: 1 0
Equil: 1 - ? n?
In the equilibrium system, the observed molar mass of the reactant is always different than the actual mass. Thus, when the reaction is reversible, the observed mass varies. In a chemical reaction, some amount of this reactant gets converted into a product, thus observed mass is different from than actual mass.
For example:
In this reaction, the original molar mass of N2O4 = 92g/mol. But the observed molar mass at equilibrium is 80g/mol. The observed molar mass is less than the original molar mass as during the reaction some amount of N2O4 is converted into NO2.
Vapour Density
Similarly, the observed density of the substance is different than the actual density.
Thus, we know:
Vapour density = Molar mass/2
Study it with Videos
Degree of Dissociation
Observed Density and Molar Mass"Stay in the loop. Receive exam news, study resources, and expert advice!"
Books
Reference Books
Clear your Basics with NCERT
E-books & Sample Papers
Get Answer to all your questions
JEE Main Articles
Back to top