Vertical Circular Motion - Practice Questions & MCQ

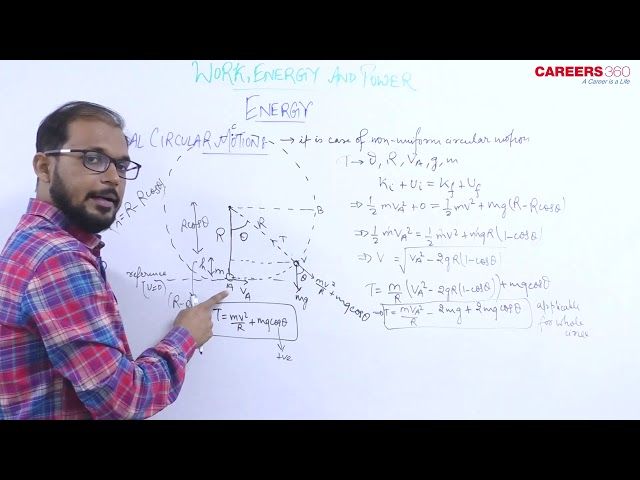
• This is an example of non-uniform circular motion.

                A particle of mass m is attached to a light and inextensible string. The other end of the string is fixed at O and

              the particle moves in a vertical circle of radius r is equal to the length of the string as shown in the figure.

• Tension at any point on the vertical loop

                                                                         

                 Consider the particle when it is at the point P and the string makes an angle θ with vertical.

                 Forces acting on the particle are:

                                T = tension in the string along its length,

                     And,  mg = weight of the particle vertically downward.

                 

Hence, the net radial force on the particle is

$
\begin{aligned}
F_r & =T-m g \cos \theta \\
F_r & =\frac{m v^2}{r}
\end{aligned}
$

Where $r=$ length of the string

$
\text { So, } \frac{m v^2}{r}=T-m g \cos \theta
$

Or, Tension at any point on the vertical loop

$
T=\frac{m v^2}{r}+m g \cos \theta
$

Since the speed of the particle decreases with height, hence, tension is maximum at the bottom, where $\cos \theta=1(\operatorname{as} \theta=0)$.

$
T_{\max }=\frac{m v_{\text {Bottom }}^2}{r}+m g
$

Similarly,

$
T_{\min }=\frac{m v_{T o p}^2}{r}-m g
$
 

• Velocity at any point on vertical loop-

                                                                         

                           If u is the initial velocity imparted to the body at the lowest point then, the velocity of the body at height h is given by

                               

$
v=\sqrt{u^2-2 g h}=\sqrt{u^2-2 g r(1-\cos \theta)}
$

- Velocity at the lowest point $(\mathrm{A})$ for the various condition in Vertical circular motion.
1. Tension in the string will not be zero at any of the point and body will continue the circular motion.

$
u_A>\sqrt{5 g r}
$

2. Tension at highest point C will be zero and body will just complete the circle.

$
u_A=\sqrt{5 g r}
$

3. A particle will not follow the circular motion. Tension in string become zero somewhere between points B and C whereas velocity remain positive. Particle leaves the circular path and follows a parabolic trajectory

$
\sqrt{2 g r}<u_A<\sqrt{5 g r}
$

4. Both velocity and tension in the string become zero between A and B and particle will oscillate along a semi-circular path.

$
u_A=\sqrt{2 g r}
$

5. The velocity of the particle becomes zero between $A$ and $B$ but the tension will not be zero and the particle will oscillate about the point A .

$
u_A<\sqrt{2 g r}
$

- Critical Velocity-

It is the minimum velocity given to the particle at the lowest point to complete the circle.

$
u_A=\sqrt{5 g r}
$