Travelling Sine Wave - Practice Questions & MCQ

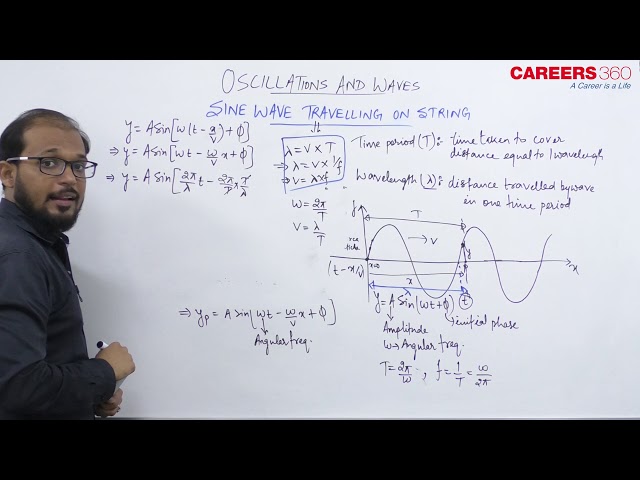
The sine wave or sinusoid is a mathematical function that describes a smooth repetitive oscillation.

$
y(t)=A \sin (\omega t+\phi)
$

Here $\omega$, is the angular frequency i.e,
$\omega=\frac{2 \pi}{T}=2 \pi f {\text { It defines how many cycles of the oscillations are there. }}$
and $\phi=$ phase angle
General form :
a spatial variable $x$ that represents the position on the dimension on which the wave propagates, and a characteristic parameter $k$ called wave number which represents the proportionality between the angular frequency $\omega$ and the linear speed (speed of propagation ) $v$.
which is $y(x, t)=A \sin (k x-\omega t+\phi)$ when the wave is moving towards the right $y(x, t)=A \sin (k x+\omega t+\phi)$ when the wave is moving towards the left.

The wavenumber is related to the angular frequency by:

$
k=\frac{\omega}{v}=\frac{2 \pi f}{v}=\frac{2 \pi}{\lambda}
$

Also,
Particle velocity $=-($ wave velocity $) \times($ slope of $y$ vs $x$ graph $)$

$
\begin{aligned}
& \Longrightarrow V_p=-v\left(\frac{\partial y}{\partial x}\right) \\
& \Longrightarrow \frac{\partial y}{\partial t}=-v\left(\frac{\partial y}{\partial x}\right)
\end{aligned}
$