Use of Integration in Binomial - Practice Questions & MCQ

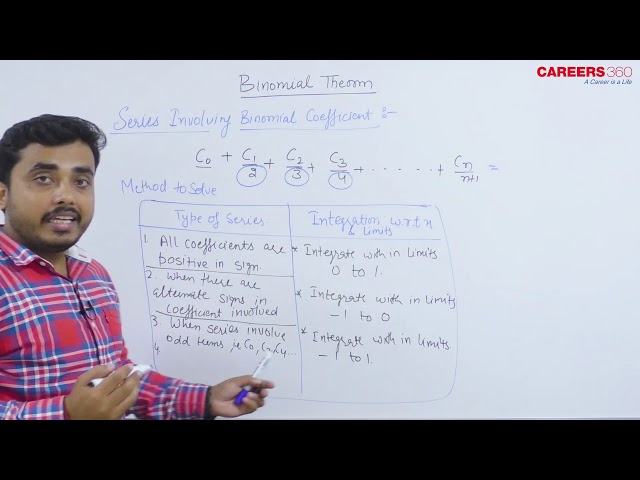
If the numbers occur as the denominator of the binomial coefficient, then this method is applicable.

Working rule:

Note:

If in the denominator of a binomial coefficient product of two numerical, then integrate two times first times taken limits between 0 to x and second times take suitable limits.

Some Binomial Series using Integration

Example

$
C_0+2^2 \cdot \frac{C_1}{2}+2^3 \cdot \frac{C_2}{3}+\ldots+2^{n+1} \cdot \frac{C_n}{n+1}=\frac{3^{n+1}-1}{n+1}
$
Proof:

$
(1+x)^n=C_0+C_1 x+C_2 x^2+\ldots .+C_n x^n
$
As each term has $+\operatorname{sign}$ and each term also has powers of 2, so we will integrate it from 0 to 2

$
\begin{aligned}
& \int_0^2(1+x)^n d x=\int_0^2\left[C_0+C_1 x+\ldots+C_n x^n\right] d x \\
& {\left[\frac{(1+x)^{n+1}}{n+1}\right]_0^2=\left[C_0 x+C_1 \cdot \frac{x^2}{2}+C_2 \cdot \frac{x^3}{3}+\ldots C_n \cdot \frac{x^{n+1}}{n+1}\right]_0^2} \\
& \Rightarrow \quad \frac{3^{n+1}-1}{n+1}=C_0 \cdot 2+2^2 \cdot \frac{C_1}{2}+2^3 \cdot \frac{C_2}{3}+\ldots+2^{n+1} \cdot \frac{C_n}{n+1}
\end{aligned}
$