Displacement Wave And Pressure Wave - Practice Questions & MCQ

Relation between displacement wave and pressure wave -

As we have studied that, when a longitudinal wave propagates in a gaseous medium, it produces rarefaction and compression in the medium, periodically. The region where compression occurs, the pressure is more as compare to the normal pressure of the medium and the region where rarefaction occurs, the pressure is lesser as compare to the normal pressure of the medium. Thus we can also describe any longitudinal waves in a gaseous medium as pressure waves and these are also termed as compressional waves.

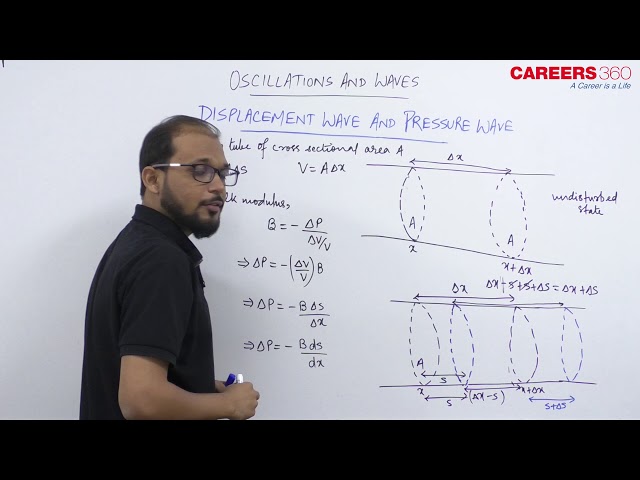
Let us consider a longitudinal wave propagating in positive 'x' direction as shown in the given figure. This figure shows a segment AB
of the medium of width 'dx'. Let a longitudinal wave is propagating in this medium whose equation is given as - 

$
y=A \sin (k x-\omega t)
$

In this equation, ' $y$ ' is the displacement of a medium particle situated at a distance ' $x$ ' from the origin along the direction of propagation of wave. From the figure, $A B$ is the medium segment such that $A$ is located at position $x=x$ and $B$ is at $x=x+d$ $x$ at an instant. If after some time $t$ medium particle at $A$ reaches to a point $A^{\prime}$ which is displaced by $y$ and the medium particle at $B$ reaches to point $B^{\prime}$ which is at a displacement $y+d y$ from $B$. Here dy is given by equation as

$
\begin{aligned}
& d y=A k \cos (k x-\omega t) d x \\
& d V=S d y=-S A k \cos (k x-\omega t) d x
\end{aligned}
$

Where, $\mathrm{S}=$ Area of cross-section and $V=$ Volume of section $A B$

$
\begin{gathered}
\frac{d V}{V}=\frac{d y}{d x}=\frac{S A k \cos (k x-\omega t) d x}{S d x} \\
\frac{d V}{V}=A k \cos (k x-\omega t)
\end{gathered}
$

If B is the bulk modulus of the medium, then the excess pressure in section $A B$ can be given as -

$
\begin{aligned}
& \Delta P=-B\left(\frac{d V}{V}\right)=-B\left(\frac{d y}{d x}\right) \\
& \Delta P=-B A k \cos (k x-\omega t) \\
& \Delta P=-\Delta P_{\max } \cos (k x-\omega t)
\end{aligned}
$

Here $\Delta P_{\max }$ is the pressure amplitude at a medium particle at position $x$ from origin
So,

$
\Delta P_{\max }=B A k=\frac{2 \pi}{\lambda} A B
$
 

In the compression zone, more particles stay in a unit volume of the medium. So, density and pressure of the region will be more. In refracted zone, lesser particles stay in any unit volume. Let a sound wave is propogating in a medium of Bulk modulus B and density .

                                                            So,

$
B=\left(-\frac{d p}{d V / V}\right)
$

Also, $\frac{d V}{V}=-\frac{d p}{p}$

From both Equation, we get, $d \rho=\frac{\rho}{B} d p$
The speed of sound is given by, $v=\sqrt{\frac{B}{\rho}} \Rightarrow \frac{\rho}{B}=\frac{1}{v^2}$ Hence, $d \rho=\frac{\rho}{B} \Delta p=\frac{1}{v^2} \Delta p$

So, this relation gives relation between pressure with density. So the variation of density is like variation of pressure -

$
\Delta \rho=(\Delta \rho)_m \sin (k x-\omega t)
$

where, $(\Delta \rho)_m=\frac{\rho}{B}(\Delta p)_m=\frac{(\Delta p)_m}{v^2}$
Note - Density equation is in phase with pressure equation and this is $\frac{\pi}{2}$ out of phase with the displacement equation