Summation Of Series In Trigonometry - Practice Questions & MCQ

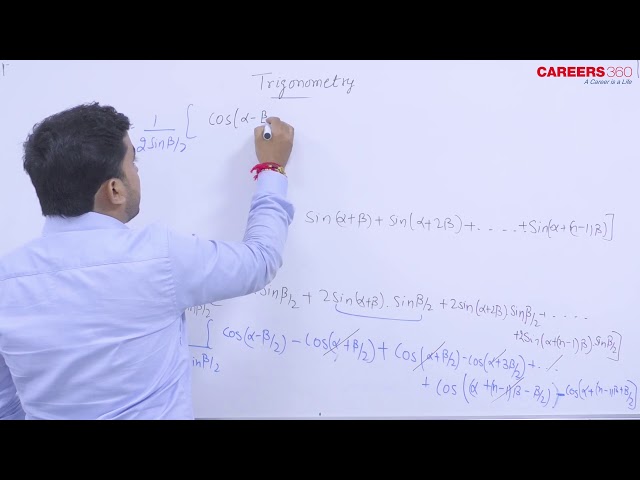
Trigonometric Series

$
\begin{gathered}
\sin (\alpha)+\sin (\alpha+\beta)+\sin (\alpha+2 \beta)+\sin (\alpha+3 \beta)+\ldots \ldots \ldots \ldots \ldots+\sin (\alpha+(n-1) \beta) \\
=\frac{\sin \left(\frac{\mathrm{n} \beta}{2}\right) \cdot \sin \left[\alpha+(\mathrm{n}-1) \frac{\beta}{2}\right]}{\sin \left(\frac{\beta}{2}\right)}
\end{gathered}
$
Proof:
Let $S=\sin \alpha+\sin (\alpha+\beta)+\sin (\alpha+2 \beta)+\cdots+\sin (\alpha+\overline{n-1} \beta)$
Here angle are in A.P. and common difference of angles $=\beta$ multiplying both side by $2 \sin \frac{\beta}{2}$ we get,

$
\begin{aligned}
& 2 S \sin \frac{\beta}{2}=2 \sin \alpha \sin \frac{\beta}{2}+2 \sin (\alpha+\beta) \sin \frac{\beta}{2}+\cdots+2 \sin (\alpha+\overline{n-1} \beta) \sin \frac{\beta}{2} \\
& \quad \text { Now, } 2 \sin \alpha \sin \frac{\beta}{2}=\cos \left(\alpha-\frac{\beta}{2}\right)-\cos \left(\alpha+\frac{\beta}{2}\right) \\
& 2 \sin (\alpha+\beta) \sin \frac{\beta}{2}=\cos \left(\alpha+\frac{\beta}{2}\right)-\cos \left(\alpha+\frac{3 \beta}{2}\right) \\
& 2 \sin (\alpha+2 \beta) \sin \frac{\beta}{2}=\cos \left(\alpha+\frac{3 \beta}{2}\right)-\cos \left(\alpha+\frac{\beta \beta}{2}\right) \\
& \ldots \\
& \cdots \\
& \cdots \\
& 2 \sin (\alpha+\overline{n-1} \beta) \sin \frac{\beta}{2}=\cos \left[\alpha+(2 n-3) \frac{\beta}{2}\right]-\cos \left[\alpha(2 n-1) \frac{\beta}{2}\right]
\end{aligned}
$

Adding all the above we get

$
\begin{aligned}
& 2 \sin \frac{\beta}{2} \mathrm{~S}=\cos \left(\alpha-\frac{\beta}{2}\right)-\cos \left(\alpha+(2 \mathrm{n}-1) \frac{\beta}{2}\right) \\
& \text { or } \\
& 2 \sin \frac{\beta}{2} \mathrm{~S}=2 \sin \left(\alpha+(\mathrm{n}-1) \frac{\beta}{2}\right) \sin \frac{\mathrm{n} \beta}{2} \\
& \Rightarrow \mathrm{~S}=\frac{\sin \left(\alpha+(\mathrm{n}-1) \frac{\beta}{2}\right) \sin \frac{\mathrm{n} \beta}{2}}{\sin \frac{\beta}{2}}
\end{aligned}
$
For cosine series

$
\cos \alpha+\cos (\alpha+\beta)+\cos (\alpha+2 \beta)+\cdots+\cos (\alpha+\overline{n-1} \beta)=\frac{\sin \frac{n \beta}{2}}{\sin \frac{\beta}{2}} \cos \left[\alpha+(n-1) \frac{\beta}{2}\right]
$