Projectile On An Inclined Plane - Practice Questions & MCQ

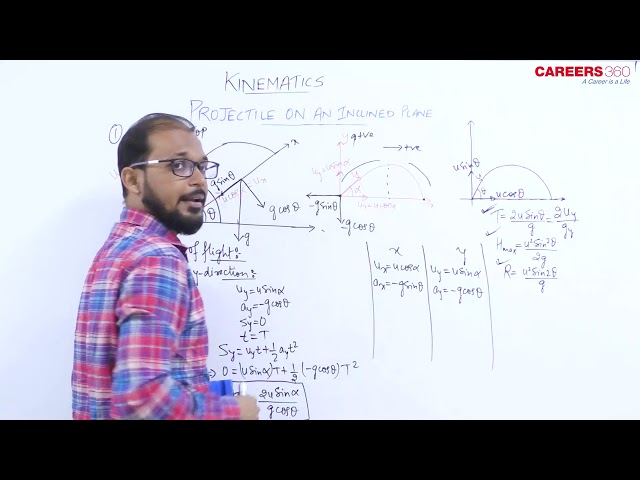
1. Important equations

                             U=Speed of projection

$\alpha=$ Angle of projection above inclined plane (measured from horizontal line)
$\theta=$ Angle of projection above inclined plane (measured from inclined plane)
$\beta=$ Angle of inclination.
a) Initial Velocity-U

Component along x or along inclined plane $=U_x=U \cos \theta$
Component along y or perpendicular to inclined plane $=U_y=U \operatorname{Sin} \theta$
b) Final velocity $=V$

Component along x or along inclined plane $=V_x=U \cos \theta-(g \sin \beta) . t$
Component along y or perpendicular to inclined plane $=V_y=U \sin \theta-(g \cos \beta) . t$
and, $V=\sqrt{V_x^2+V_y^2}$
c) Displacement=S

Component along x or along inclined plane $=S_x=U_x t+\frac{1}{2} a_x \cdot t^2$
Component along y or perpendicular to inclined plane $=S_y=U_y t+\frac{1}{2} a_y \cdot t^2$
And $S=\sqrt{S_x^2+S_y^2}$
d) Acceleration = a

Component along x or along inclined plane $=a_x=-g \sin \beta$

    Component along y or perpendicular to  inclined plane =

    So a=-g

2.  Important Terms
a) Time of flight

Formula

$
T=\frac{2 U \sin \theta}{g \cos \beta}
$

b) Range along incline plane

Formula

$
R=\frac{2 u^2 \cdot \sin (\alpha-\beta) \cdot \cos \alpha}{g \cos ^2 \beta}
$