Orbital Velocity - Practice Questions & MCQ

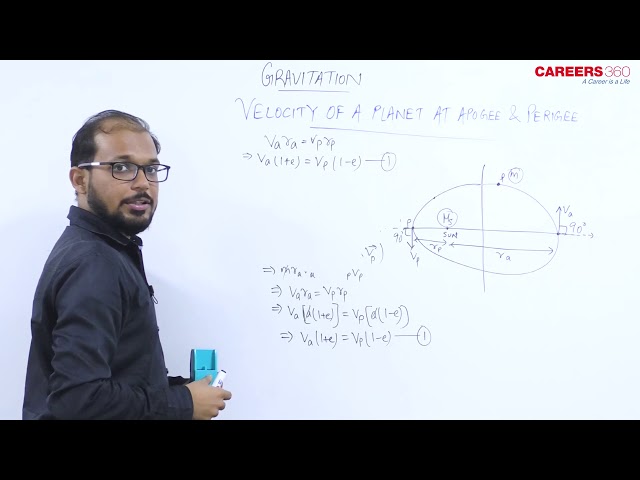
For the below figure

$
\begin{aligned}
& r_1=r_p=a-c \\
& r_2=r_a=a+c
\end{aligned}
$

If Eccentricity is given by

$
\text { (e) }=\frac{c}{a}
$

then The velocity of the planet at Apogee and Perigee in terms of Eccentricity is given by

$
\begin{aligned}
V_a & =\sqrt{\frac{G M}{a}\left(\frac{1-e}{1+e}\right)} \\
V_p & =\sqrt{\frac{G M}{a}\left(\frac{1+e}{1-e}\right)} \\
V_A & =\text { The velocity of the planet at apogee } \\
V_p & =\text { Velocity of perigee }
\end{aligned}
$

Proof-
Let the mass of the sun be $M$ and mass of the planet be $m$
Applying the law of conservation of angular momentum at perigee and apogee about the sun

$
\begin{aligned}
& m v_p r_p=m v_a r_a \\
\Rightarrow & \frac{v_p}{v_a}=\frac{r_a}{r_p}=\frac{a+c}{a-c}=\frac{1+e}{1-e}
\end{aligned}
$
 

Applying the conservation of mechanical energy at perigee and apogee

$
\begin{aligned}
& \frac{1}{2} m v_p^2-\frac{G M m}{r_p}=\frac{1}{2} m v_a^2-\frac{G M m}{r_a} \Rightarrow v_p^2-v_a^2=2 G M\left[\frac{1}{r_p}-\frac{1}{r_a}\right] \\
\Rightarrow & v_a^2\left[\frac{r_a^2-r_p^2}{r_p^2}\right]^2=2 G M\left[\frac{r_a-r_p}{r_a r_p}\right] \quad\left[\text { As } v_p=\frac{v_a r_a}{r_p}\right]
\end{aligned}
$

Applying the conservation of mechanical energy at perigee and apogee

$
\begin{aligned}
& \frac{1}{2} m v_p^2-\frac{G M m}{r_p}=\frac{1}{2} m v_a^2-\frac{G M m}{r_a} \Rightarrow v_p^2-v_a^2=2 G M\left[\frac{1}{r_p}-\frac{1}{r_a}\right] \\
\Rightarrow & v_a^2\left[\frac{r_a^2-r_p^2}{r_p^2}\right]^2=2 G M\left[\frac{r_a-r_p}{r_a r_p}\right] \quad\left[\text { As } v_p=\frac{v_a r_a}{r_p}\right] \\
\Rightarrow & v_a^2=\frac{2 G M}{r_a+r_p}\left[\frac{r_p}{r_a}\right] \Rightarrow v_a^2=\frac{2 G M}{2 a}\left(\frac{a-c}{a+c}\right)=\frac{G M}{a}\left(\frac{1-e}{1+e}\right)
\end{aligned}
$

Thus the speeds of planet at apogee and perigee are

$
v_a=\sqrt{\frac{G M}{a}\left(\frac{1-e}{1+e}\right)}, \quad v_p=\sqrt{\frac{G M}{a}\left(\frac{1+e}{1-e}\right)}
$